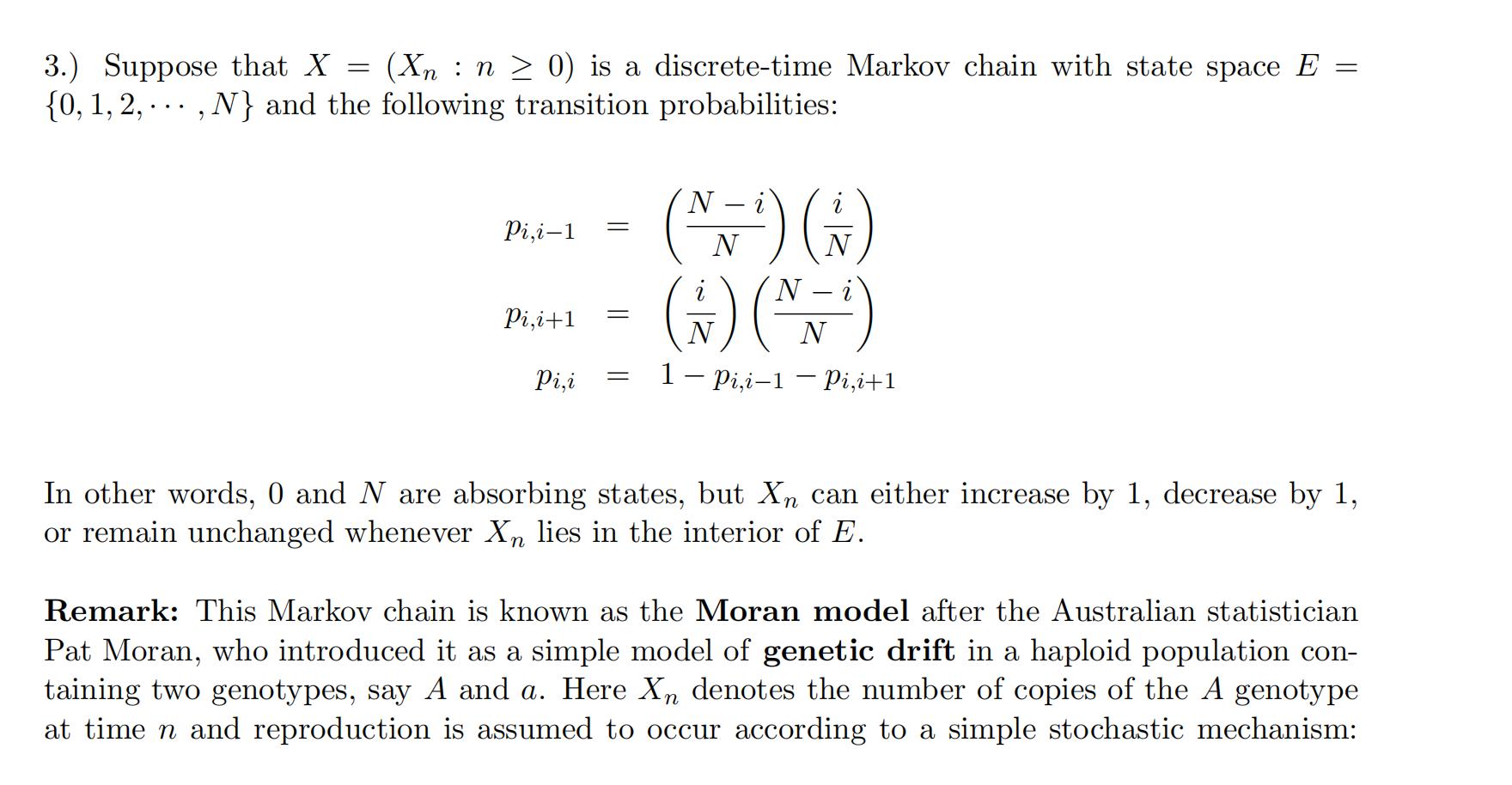
3.) Suppose that X (Xn: n 0) is a discrete-time Markov chain with state space E = {0, 1, 2,.,N} and the following transition probabilities: = Pi,i-1 - Pi,i+1 = Pi,i = i (N) (+) N (+) (+) N 1 - Pi,i-1 - Pi,i+1 In other words, 0 and N are absorbing states, but Xn can either increase by 1, decrease by 1, or remain unchanged whenever Xn lies in the interior of E. Remark: This Markov chain is known as the Moran model after the Australian statistician Pat Moran, who introduced it as a simple model of genetic drift in a haploid population con- taining two genotypes, say A and a. Here Xn denotes the number of copies of the A genotype at time n and reproduction is assumed to occur according to a simple stochastic mechanism: at each time, two individuals are sampled at random from the population. One of these indi- viduals reproduces, giving birth to a single offspring that inherits the parental genotype, while the other individual dies. Because birth and death events are coupled, the population size N remains constant. However, the numbers of copies of the two genotypes can change at random, ultimately leading to loss of one genotype and fixation of the other. For the purposes of this problem, let us assume that N = 100 and that Xo = 50. (a) Calculate or estimate the mean and the variance of X40. (b) Calculate or estimate the probability that X40 {0, 100}, i.e., one of the two alleles has been fixed in the population, while the other allele has been lost. You can answer these questions using either Monte Carlo simulations or numerical linear algebra, but please provide a copy of your code with your solutions.