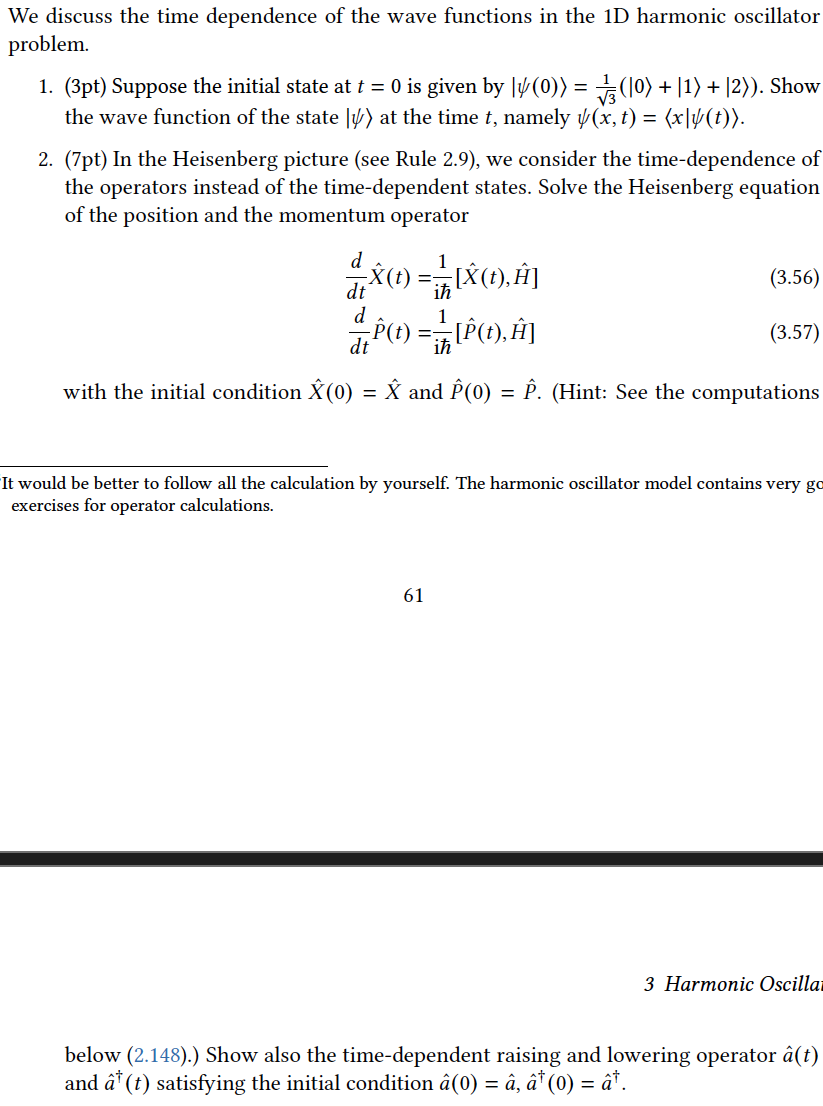
We discuss the time dependence of the wave functions in the 1D harmonic oscillator problem. 1. (3pt) Suppose the initial state at t = 0 is given by |1 (0)) = 13 (10) + |1) + |2)). Show the wave function of the state |) at the time t, namely y(x,t) = (x|y(t)). 2. (7pt) In the Heisenberg picture (see Rule 2.9), we consider the time-dependence of the operators instead of the time-dependent states. Solve the Heisenberg equation of the position and the momentum operator d dt x(t) = [ (t), ] d i 1 +P(1) = [P(1), ] (3.56) (3.57) dt with the initial condition (0) = and (0) = . (Hint: See the computations It would be better to follow all the calculation by yourself. The harmonic oscillator model contains very go exercises for operator calculations. 61 3 Harmonic Oscilla below (2.148).) Show also the time-dependent raising and lowering operator (t) and a* (t) satisfying the initial condition (0) = , (0) = *.