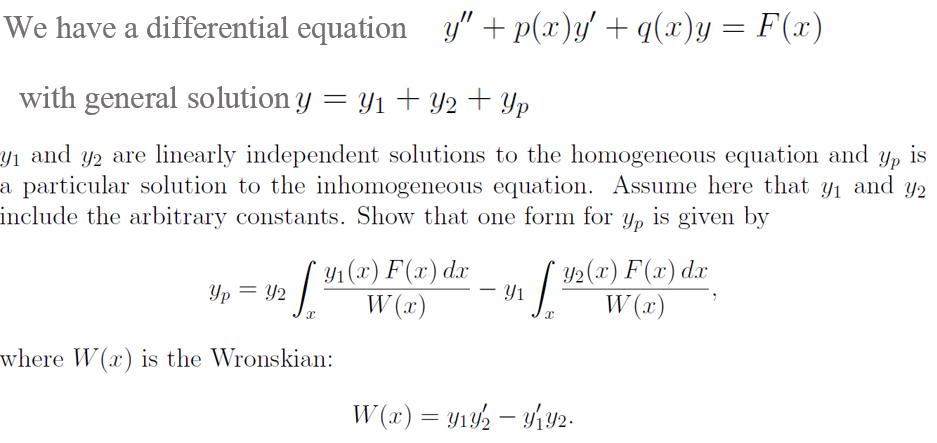
We have a differential equation y p(x)y' q(x)y F(x) with general solution y y y2 Yp y and y2 are linearly independent solutions to the homogeneous equation and a particular solution to the inhomogeneous equation Assume here that Y1 and Y2 include the arbitrary constants Show that one form for Yp i...

Given differential equation is ypxyqxyFx 0 the complementary solution to i is ...

We have a differential equation y + p(x)y' +q(x)y = F(x) with general solution y =...

Fantastic news! We've Found the answer you've been seeking!

Question:

Related Book For answer-question

A First Course in Differential Equations with Modeling Applications

ISBN: 978-1111827052

10th edition

Authors: Dennis G. Zill

See More Books

Posted Date: Sep 28, 2022 02:14 AM

See More Questions

Show that if u and v are linearly independent vectors, then so are u + v and u - v.
The demand for sunglasses is given by equation Qd = 1000 - 4P, where P denotes the market price. The supply of sunglasses is given by equation Qs = 100 + 6P. Fill in the following table and find the...
Assume that the demand curve for milk is given by Q = 100 - 10 P , where P is the price per gallon and Q is the quantity demanded per year. The supply curve is horizontal at a price of 2. a. Assuming...
All else equal, a large sell off in the equity markets (decline in the S&P 500 and the Dow Jones Industrial Average) would be the most likely result from the reporting of a much higher than expected...
Sonya Kaminski purchased from Billy Cains Cornelia dealership a truck that was represented to her to be a 1989 Chevrolet Silverado pickup. However, subsequent incidents involving repair of the truck...
Consider the broad shifts in marketing. Do any themes emerge in them? Can you relate the shifts to the major societal forces? Which force has contributed to which shift?
Chris Zarb, the marketing manager for Maltese Treasures Ltd, is preparing a sales budget for the year ended 30 June 2025. In reviewing the actual sales data for the previous year, the sales and...
Charles Brandon is thinking about investing in the Purple Arrow Company, which makes central air conditioning units. The company has been in business for over 50 years, and Charles believes it is...
Prepare a physical unit flow reconciliation with the following information. Units of Blending Process Beginning work in process inventory Units started this period Product 182,000 390,000 Units...
Cullumber Warehouse distributes hardback books to retail stores and extends credit terms of 4/10, n/30 to all of its customers. During the month of June, the following merchandising transactions...
What is a Distributed File System (DFS)? How do DFSs work? Compare and contrast DFS technology with describe about storing data in a Data Warehouse
Calculate the magnitude of the average current in the wire loop the magnet is falling. A bar magnet is held above a wire loop and dropped. The loop has a diameter of 5.5cm Immediately before...
Raul is more likely to wear a sweater to school when the temperature outside is 32 degrees than when it is 82 degrees. What is the correlation between the temperature outside and Raul's choice of...
In March, Stinson Company completes Jobs 10 and 11. Job 10 cost $25,500 and Job 11 $38,000. On March 31, Job 10 is sold to the customer for $38,000 in cash. Journalize the entries for the completion...
Make a presentation question The Case-Based Procurement Project focuses on designing an effective procurement strategy for a hypothetical company operating in Canada. The primary objective of this...
Please provide the answer of the question: P Cost of production (2010 US$ per barrel of oil) 110 100 Conventional 2P reserves in production or scheduled 0 Reserve growth Undiscovered Arctic 90 Light...
You boot up a machine to start a forensic investigation. You get a message on screen indicating that the "Master Boot Record Cannot Be Found." What step of the boot process has failed? POST has...
(a) Prove that form an orthonormal basis for R3 for the usual dot product. (b) Find the coordinates of v = (1, 1, 1)T relative to this basis. (c) Verify formula (5.5) in this particular case. 48-65...
In problem use the procedures developed in this chapter to find the general solution of each differential equation. y'' - 2y' + 2y = e x tan x
In problem, x = 0 is a regular singular point of the given differential equation. Show that the indicial roots of the singularity differ by an integer. Use the method of Frobenius to obtain at least...
In problem use appropriate algebra and Theorem 7.2.1 to find the given inverse Laplace transform. Theorem 7.2.1 s - 3 V3)(s + V3)
Describe which characteristics of HR metrics and workforce analytics are likely to result in greater return on investment and organizational impact.
Why are information security and privacy important considerations in the design, development, and maintenance of an HRIS?
List and discuss the major information security and privacy threats to organizations.

We have a differential equation y + p(x)y' +q(x)y = F(x) with general solution y =...

Question:

Expert Answer:

Given differential equation is ypxyqxyFx 0 the complementary solution to i is ... View the full answer

A First Course in Differential Equations with Modeling Applications

Students also viewed these accounting questions