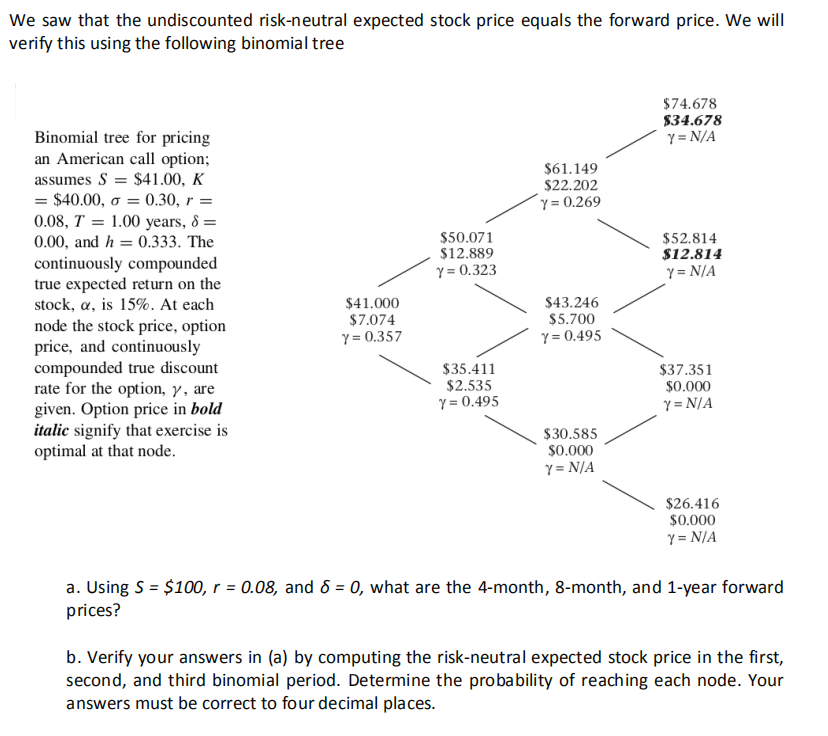
We saw that the undiscounted risk-neutral expected stock price equals the forward price. We will verify this using the following binomial tree $74.678 $34.678 y =N/A $61.149 $22.202 y = 0.269 $50.071 $12.889 y = 0.323 $52.814 $12.814 7 = N/A Binomial tree for pricing an American call option; assumes S = $41.00, K = $40.00, 0 = 0.30, r = 0.08, T = 1.00 years, 8 = 0.00, and h = 0.333. The continuously compounded true expected return on the stock, a, is 15%. At each node the stock price, option price, and continuously compounded true discount rate for the option, y, are given. Option price in bold italic signify that exercise is optimal at that node. $41.000 $7.074 y = 0.357 $43.246 $5.700 y = 0.495 $35.411 $2.535 y = 0.495 $37.351 $0.000 Y=N/A $30.585 $0.000 Y = N/A $26.416 $0.000 Y = N/A a. Using S = $100, r = 0.08, and 8 = 0, what are the 4-month, 8-month, and 1-year forward prices? b. Verify your answers in (a) by computing the risk-neutral expected stock price in the first, second, and third binomial period. Determine the probability of reaching each node. Your answers must be correct to four decimal places. We saw that the undiscounted risk-neutral expected stock price equals the forward price. We will verify this using the following binomial tree $74.678 $34.678 y =N/A $61.149 $22.202 y = 0.269 $50.071 $12.889 y = 0.323 $52.814 $12.814 7 = N/A Binomial tree for pricing an American call option; assumes S = $41.00, K = $40.00, 0 = 0.30, r = 0.08, T = 1.00 years, 8 = 0.00, and h = 0.333. The continuously compounded true expected return on the stock, a, is 15%. At each node the stock price, option price, and continuously compounded true discount rate for the option, y, are given. Option price in bold italic signify that exercise is optimal at that node. $41.000 $7.074 y = 0.357 $43.246 $5.700 y = 0.495 $35.411 $2.535 y = 0.495 $37.351 $0.000 Y=N/A $30.585 $0.000 Y = N/A $26.416 $0.000 Y = N/A a. Using S = $100, r = 0.08, and 8 = 0, what are the 4-month, 8-month, and 1-year forward prices? b. Verify your answers in (a) by computing the risk-neutral expected stock price in the first, second, and third binomial period. Determine the probability of reaching each node. Your answers must be correct to four decimal places