Answered step by step
Verified Expert Solution
Question
1 Approved Answer
We shall consider the function h given by h(n)=lg(n!) (for n>1). That is, (1) TL h(n)=lg(i)= =lg( lg(i) i=1 i=1 Prove, from the definition
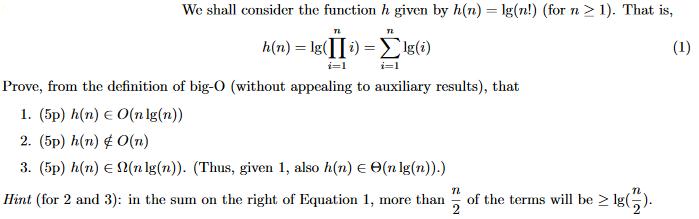
We shall consider the function h given by h(n)=lg(n!) (for n>1). That is, (1) TL h(n)=lg(i)= =lg( lg(i) i=1 i=1 Prove, from the definition of big-O (without appealing to auxiliary results), that 1. (5p) h(n) O(ng(n)) 2. (5p) h(n) 3. (5p) h(n) O(n) (n lg(n)). (Thus, given 1, also h(n) (n Ig(n)).) 72 Hint (for 2 and 3): in the sum on the right of Equation 1, more than of the terms will be > Ig().
Step by Step Solution
There are 3 Steps involved in it
Step: 1
The image youve provided contains a question from a mathematical or computer science context regarding BigO notation which is used to classify algorit...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Elements Of Chemical Reaction Engineering
Authors: H. Fogler
6th Edition
013548622X, 978-0135486221
