We assume that g(x) is a function defined on (0, 100) and has a derivative g'(x) and a primitive...

Transcribed Image Text:

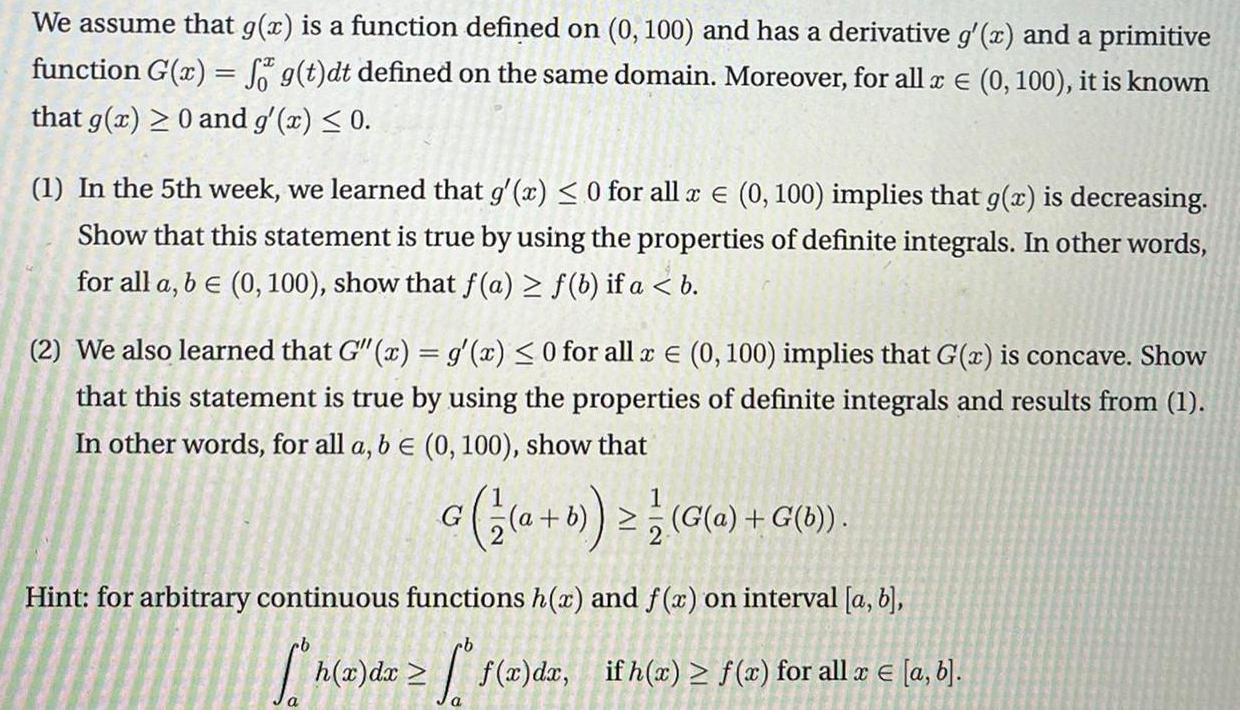
We assume that g(x) is a function defined on (0, 100) and has a derivative g'(x) and a primitive function G(x) = g(t)dt defined on the same domain. Moreover, for all x € (0, 100), it is known that g(x) > 0 and g'(x) ≤ 0. (1) In the 5th week, we learned that g'(x) ≤ 0 for all x € (0, 100) implies that g(x) is decreasing. Show that this statement is true by using the properties of definite integrals. In other words, for all a, b € (0, 100), show that f(a) ≥ f(b) if a < b. (2) We also learned that G"(x) = g'(x) ≤ 0 for all x € (0, 100) implies that G(x) is concave. Show that this statement is true by using the properties of definite integrals and results from (1). In other words, for all a, b € (0, 100), show that G (12(a + b)) ≥ 1/ (G(a) + G(b)). Hint: for arbitrary continuous functions h(x) and f(x) on interval [a, b], ["h(x)dx ≥ ſª f(x)dx, _ifh(x) ≥ ƒ(x) for all x = [ª, b]. a

Answer rating: 100% (QA)