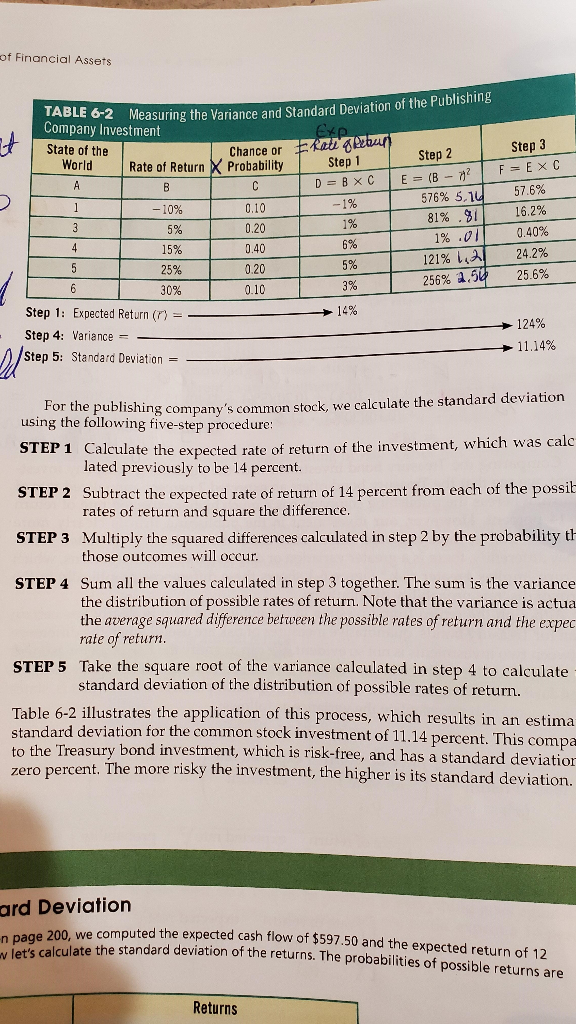
When trying to calculate step 2 and step 3 in the table 6.2 below (see the pic below), if I convert the %'s to decimals or leave them as %'s why are my numbers so far off? When calculating variances should I not convert to the percentages to decimals?. For example - when I calculate the variance for state 1 if r = 14%. This is how I would calculate it and it doesn't even work in Excel. Also, is there a formula in Excel to calculate these variances? I cannot seem to find one.

-10%-14%= -24%

-24% squared = 5.76% variance. (The book has the variance as 576%)

If I instead perform the same calculations and convert everything to decimals in my calculations, here is what I get:

-.10-.14= -0.24

-0.24 squared = .0576 or 5.76% variance.

of Financial Assets EX Step 2 E = (B - 72 576% 5.14 Step 3 F = EXC 57.6% 16.2% 0.40% 1% .01 121% lach 256% 2.54 TABLE 6-2 Measuring the Variance and Standard deviation of the Publishing Company Investment State of the Chance or Ekau guztur World Rate of Return X Probability Step 1 A B D = B XC 1 -10% 0.10 - 1% 81% .81 3 5% 0.20 1% 4 15% 0.40 6% 24.2% 5 25% 0.20 5% 25.6% 30% 0.10 3% Step 1: Expected Return (7) 14% 124% Step 4: Variance = 11.14% Step 5: Standard deviation - For the publishing company's common stock, we calculate the standard deviation using the following five-step procedure: STEP 1 Calculate the expected rate of return of the investment, which was calc lated previously to be 14 percent. STEP 2 Subtract the expected rate of return of 14 percent from each of the possit rates of return and square the difference. STEP 3 Multiply the squared differences calculated in step 2 by the probability tH those outcomes will occur. STEP 4 Sum all the values calculated in step 3 together. The sum is the variance the distribution of possible rates of return. Note that the variance is actua the average squared difference between the possible rates of return and the expec rate of return STEP 5 Take the square root of the variance calculated in step 4 to calculate standard deviation of the distribution of possible rates of return. Table 6-2 illustrates the application of this process, which results in an estima standard deviation for the common stock investment of 11.14 percent. This compa to the Treasury bond investment, which is risk-free, and has a standard deviatior zero percent. The more risky the investment, the higher is its standard deviation. ard Deviation n page 200, we computed the expected cash flow of $597.50 and the expected return of 12 let's calculate the standard deviation of the returns. The probabilities of possible returns are Returns