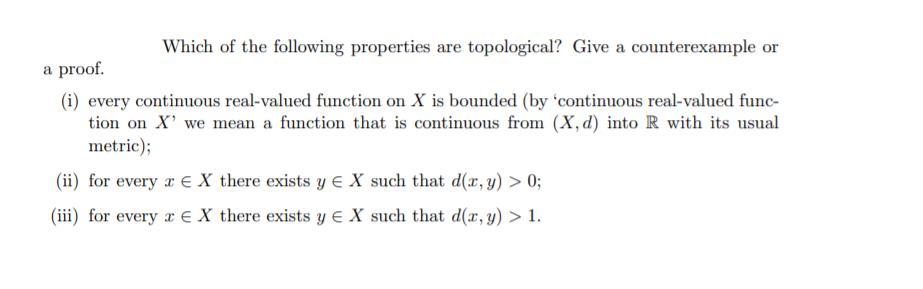
Which of the following properties are topological? Give a counterexample or a proof. (i) every continuous real-valued function on X is bounded (by 'continuous real-valued func- tion on X' we mean a function that is continuous from (X, d) into R with its usual metric); (ii) for every r E X there exists y E X such that d(r, y) > 0; (iii) for every r E X there exists y E X such that d(x, y) > 1.