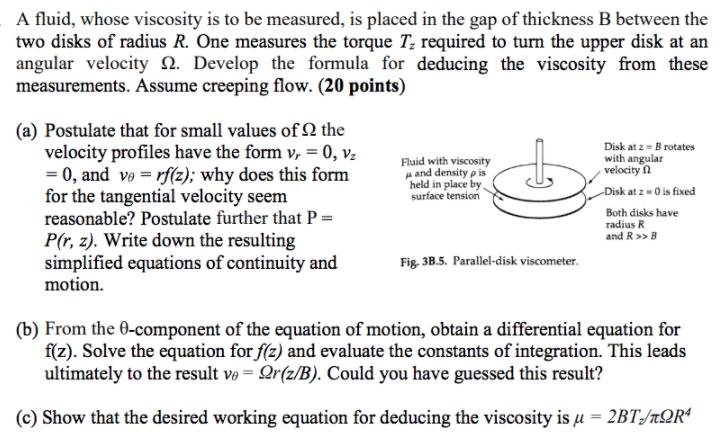
A fluid, whose viscosity is to be measured, is placed in the gap of thickness B between the two disks of radius R. One measures the torque T; required to turn the upper disk at an angular velocity 2. Develop the formula for deducing the viscosity from these measurements. Assume creeping flow. (20 points) (a) Postulate that for small values of Q the velocity profiles have the form v, = 0, vz = 0, and vo = rf(z); why does this form for the tangential velocity seem reasonable? Postulate further that P= P(r, z). Write down the resulting simplified equations of continuity and motion. Disk at z-B rotates with angular velocity n Fluid with viscosity u and density p is held in place by surface tension Disk at z-0 is fixed Both disks have radius R and R>> B Fig, 3B.5. Parallel-disk viscometer. (b) From the 0-component of the equation of motion, obtain a differential equation for f(z). Solve the equation for f(z) and evaluate the constants of integration. This leads ultimately to the result ve = Or(z/B). Could you have guessed this result? (c) Show that the desired working equation for deducing the viscosity is u = 2BT/rQR*