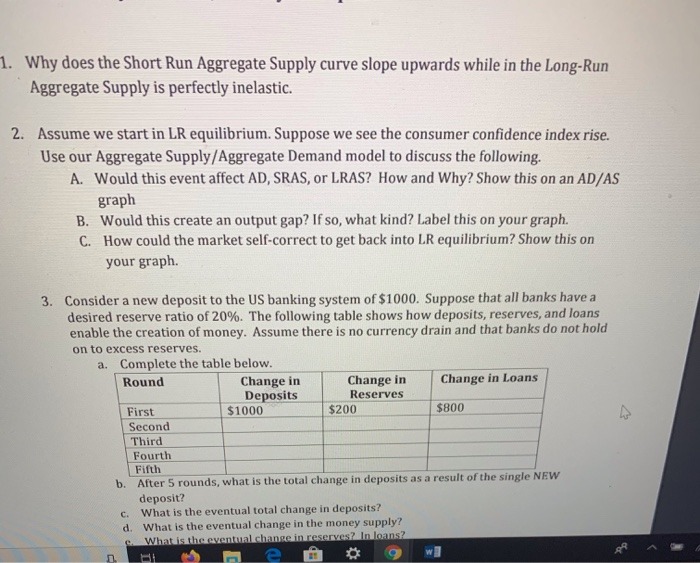
Why does the Short Run Aggregate Supply curve slope upwards while in the Long-Run Aggregate Supply is perfectly inelastic. 2. Assume we start in LR equilibrium. Suppose we see the consumer confidence index rise. Use our Aggregate Supply/Aggregate Demand model to discuss the following. A. Would this event affect AD, SRAS, or LRAS? How and Why? Show this on an AD/AS graph B. Would this create an output gap? If so, what kind? Label this on your graph. C. How could the market self-correct to get back into LR equilibrium? Show this on your graph. 3. Consider a new deposit to the US banking system of $1000. Suppose that all banks have a desired reserve ratio of 20%. The following table shows how deposits, reserves, and loans enable the creation of money. Assume there is no currency drain and that banks do not hold on to excess reserves. a. Complete the table below. Round Change in Change in Change in Loans Deposits Reserves First $1000 $200 $800 Second Third Fourth Fifth b. After 5 rounds, what is the total change in deposits as a result of the single NEW deposit? C. What is the eventual total change in deposits? d. What is the eventual change in the money supply? What is the eventual change in reserves? In loans?11. Suppose the demand for real money balances is M"/P = L(Y, i), where L(Y, /) is an increasing function of income Y and a decreasing function of the nominal inter- est rate i. Assume that the interest elasticity of money demand is infinite when the nominal interest rate is zero. Money-market equilibrium is represented by the equation MS/P = L(Y, /), where M' is the money supply controlled by the central bank and P is the price level. The LM curve relationship between Y and i repre- sents money-market equilibrium for given values of M' and P. In the IS-LM model. the price level P is assumed to be fixed in the short run. (a) [5 marks] Show how the LM curve is derived, paying careful attention to its shape where / is zero. The IS curve represents goods-market equilibrium. Consumption depends posi- tively on disposable income; investment depends negatively on the real interest rate; tax and government spending are exogenous. Following a large negative shock to confidence, investment demand falls and the IS curve shifts to the left, intersecting the LM curve at / = 0. (b) [8 marks] Use the IS-LM model to analyse the effects on GDP of each of the following policy options. Which policies does the model predict are effective in avoiding a recession? i. The central bank increases the money supply by buying more short-term government bonds ('quantitative easing); ii. Higher government expenditure paid for by increasing taxes; iii. A tax cut paid for by printing money (a helicopter drop' of money); iv. The central bank raises its inflation target (assume this is credible and increases inflation expectations ,"). Now consider Tobin's model of the speculative demand for money. Risk-averse investors can hold money or long-term bonds. Money is a safe asset, but offers a zero return. Long-term bonds have a positive expected return, but are risky assets. (c) [3 marks] Explain intuitively how investors determine the shares of long-term bonds and money in their portfolios. For a given level of riskiness of long- term bonds, what happens to bond demand if the bond yield falls? (d) [4 marks] Assume that the central bank implements quantitative easing by buying long-term bonds with a positive yield rather than zero-yielding short- term bonds. According to Tobin's model, what must happen to long-term bond yields for the bond market to be in equilibrium? Explain.10. Consider a government with preferences represented by the loss function L = 72 + au2 where it is the inflation rate, u is the unemployment rate, and a is a positive con- stant indicating the strength of the government's preference for low and stable unemployment relative to low and stable inflation. The expectations-augmented Phillips curve linking inflation and unemployment is: U = Un - b(7 - 7) where " denotes inflation expectations, Un is the natural rate of unemployment, and b is a positive constant. Expectations of inflation are assumed to be rational. Assume that monetary policy is able to control the inflation rate it directly. Suppose initially that the government chooses a monetary policy without being restricted by any past commitments (the government acts with discretion). This means inflation expectations ," are taken as given when monetary policy is cho- sen. (a) [3 marks] Derive the first-order condition for the inflation rate ? that mini- mizes the loss function subject to the Phillips curve for given ?". As expectations are formed rationally, everyone anticipates the actions of the cen- tral bank determined in part (a). (b) [3 marks] Find the equilibrium unemployment and inflation rates in terms of a, b, and Un. (c) [4 marks] Suppose political pressure forces the government to shift its focus away from inflation towards unemployment. Interpreting this as an increase in a in the loss function, what are the effects on the equilibrium values of u and ? Explain your findings with reference to the policy ineffectiveness proposition. The government announces it will follow a rule that strictly targets inflation . (d) [4 marks] Assuming that everyone believes the rule will be followed, find the optimal rule for the inflation rate that minimizes the loss function. What is the equilibrium unemployment rate when the government follows the rule? (e) [6 marks] Once everyone chooses inflation expectations consistent with your answer to part (d), suppose the government is now free to choose inflation taking a" as given as it did in part (a). Solve for the resulting values of u and ". By comparing your answer to part (d), explain why the optimal rule for inflation is time inconsistent.1. What was the path of the money stock in the U.S. from January 1929 to March 1933? How did household's holdings of currency change over the same period? 2. What was the path of real income in the U.S. from January 1929 to March 1933? How did prices change over the same period? 3. In the period 1948-1991 have American real interest rates ever been negative? In the same period, has the U.S. inflation rate ever been negative? If so, when? 4. What is the evidence that inflation, in Milton Friedman's words, "is always and ev- erywhere a monetary phenomenon"? In the long run? In the short run? 5. If you take the population of the U.S. to be 260 millions, roughly how many dollars of currency were in circulation for every U.S. citizen at the end of 1995? How much currency are you carrying right now? How do you account for this discrepancy? 6. Why do people hold currency and keep part of their wealth in low interest bearing accounts (like the Hyde Park Bank's zero interest checking account)? 7. Explain how a rational expectations view of agent's behavior (as defined by Sargent) can explain why inflation seems to have momentum, while in fact it does not. 8. What is seignorage? How much money did the U.S. raise via seignorage in 1991? 9. What is the Quantity Theory of Money? Explain the sense in which it is "just" an accounting identity. 10. What is a gold standard? True or false: Under a gold standard the quantity of money is fixed. Exercise 18.2 (Easy) Evaluate this statement: Government austerity programs cause civil unrest. Exercise 18.3 (Moderate) In each period t the government raises real tax revenue of 7, and spends (in real terms) Gr. Let D, = Gr -T, be the real deficit at time t. At the suggestion of a revered elder whose initials are M.F., the government is allowed to finance this deficit only by issuing fiat currency and obtaining the seignorage revenue. The government's budget constraint is thus: D, = Alt - Mi-1 where D, is the real government deficit att, M, is the stock of money at t and P, is the price level at t. Prices are related to the money supply by the Quantity Theory of Money relation with a constant velocity v = 1: PIV, = M. Exercises 225 Output Y, satisfies Y, = M, where N, is population att, and evolves according to: N = (1 + n)N-1, with No = 1. The government runs a constant per capita real deficit of d, so D, = dN, for all t. Answer the following questions: 1. How must M evolve given Mr- and d? 2. For what value of d is the inflation rate zero? That is, for what value of d will Pr = Pi-1? 3. A reasonable estimate for n is about 0.03. At this value, how large a deficit, expressed as a fraction of GDP, can the government cover by printing money and still not cause inflation? 4. Assumed = 0. What happens to prices?Exercises Exercise 1.1 (Easy) My credit card has an APR (annualized percentage rate) of 16.8%. What is the daily interest rate? Exercise 1.2 (Easy) My loan shark is asking for $25 in interest for a one-week loan of $1,000. What is that, as an annual interest rate? (Use 52 weeks per year.) Exercise 1.3 (Moderate) The Consumer Price Index (CPD) is a measure of the prices of goods that people buy. Bigger numbers for the index mean that things are more expensive. Here are the CPI numbers for four months of 1996 and 1997: 6 Preliminaries Variable Definition Principal (amount invested) R Nominal interest rate Number of years invested V,(n) Value after n years under simple interest Va(n) Value after n years under annual compounding Vi(n) Value after n years when compounded t times per year Ve(n) Value after n years under continuous compound- ing Vo Initial value of the investment Table 1.1: Notation for Chapter 1 Year | Mar Jun Sep Dec 1996 155.7 156.7 157.8 158.6 7 160.0 160.3 161.2 161.3 What is the growth rate of the CPI between June 1996 and September 1996? (Use a contin- uous growth rate and annualize your answer.) Exercise 1.4 (Moderate) Use the CPI data from the previous exercise to compute the growth rates in the CPI in the four quarters starting in March 1996 (i.e, Mar-Jun 1996, Jun-Sep 1996, etc.). (Use a continuous growth rate but do not annualize your answer.) Show that the sum of these four rates equals the (continuous) growth rate from March 1996 to March 1997. Exercise 1.5 (Easy) Real output of the United States will likely grow by about 2% over the first half of the next century. At that rate (of continuous growth), how long will it take for real output to double? Compare your exact answer with the approximation given by the "Rule of 72." Exercise 1.6 (Hard) This morning you invest $10,000 at 6.5% interest that compounds annually. What is the first date on which you would have at least $15,000? (Quote the answer in terms of years + days from today. Interest accrues each night, but compounds only annually.) Exercise 1.7 (Easy) Suppose that 4.6 percent of the earth's forests are cleared each year. How long will it take "The "Rule of 72" is as follows. If the interest rate on an investment is a percent, then it takes about 72/r years for the value of the investment to double