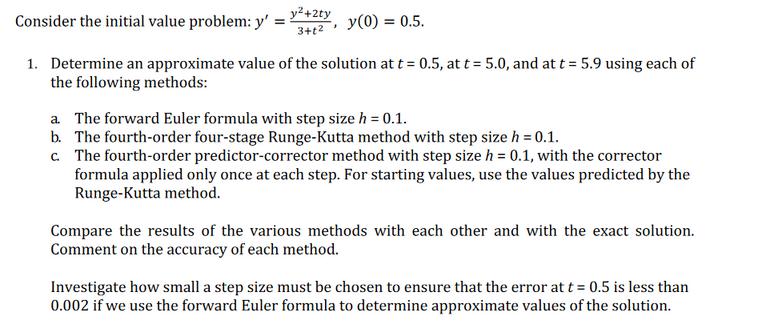
y+2ty 3+t Consider the initial value problem: y' y (0) = 0.5. 1. Determine an approximate value of the solution at t = 0.5, at t = 5.0, and at t = 5.9 using each of the following methods: a. The forward Euler formula with step size h = 0.1. b. The fourth-order four-stage Runge-Kutta method with step size h = 0.1. c. The fourth-order predictor-corrector method with step size h = 0.1, with the corrector formula applied only once at each step. For starting values, use the values predicted by the Runge-Kutta method. Compare the results of the various methods with each other and with the exact solution. Comment on the accuracy of each method. Investigate how small a step size must be chosen to ensure that the error at t = 0.5 is less than 0.002 if we use the forward Euler formula to determine approximate values of the solution.