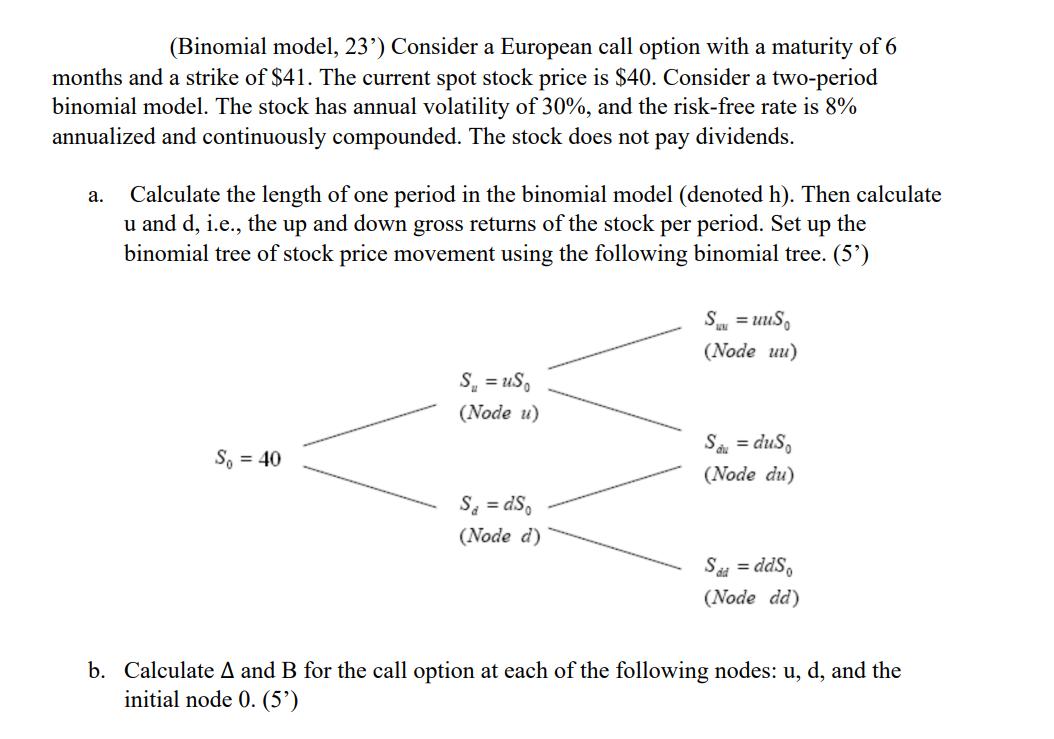
(Binomial model, 23') Consider a European call option with a maturity of 6 months and a strike of $41. The current spot stock price is $40. Consider a two-period binomial model. The stock has annual volatility of 30%, and the risk-free rate is 8% annualized and continuously compounded. The stock does not pay dividends. a. Calculate the length of one period in the binomial model (denoted h). Then calculate u and d, i.e., the up and down gross returns of the stock per period. Set up the binomial tree of stock price movement using the following binomial tree. (5') So = 40 S = US (Node u) S = dSo (Node d) S = 1 uus (Node uu) Sdu = dus (Node du) S dd = ddSo (Node dd) b. Calculate A and B for the call option at each of the following nodes: u, d, and the initial node 0. (5') c. Calculate the value of the call option at each of the nodes. (Hint: Use the formula: C = ASi + B, where i denotes node 0, u, or d) (3) d. What is a self-financing strategy? Discuss why your replicating strategy in (b) and (c) is self-financing at nodes u and d. (5') e. Calculate the risk-neutral probability at each node of u, d, and the initial node 0. Calculate the call option price using the risk-neutral probability method. (5')