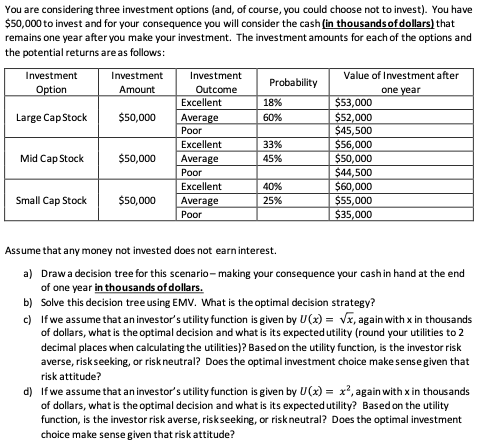
You are considering three investment options (and, of course, you could choose not to invest). You have $50,000 to invest and for your consequence you will consider the cash (in thousands of dollars) that remains one year after you make your investment. The investment amounts for each of the options and the potential returns are as follows: Investment Option Investment Amount Probability 18% 60% Large Cap Stock $50,000 Investment Outcome Excellent Average Poor Excellent Average Poor Excellent Average Poor 33% 45% Value of Investment after one year $53,000 $52,000 $45,500 $56,000 $50,000 $44,500 $60,000 $55,000 $35,000 Mid Cap Stock $50,000 Small Cap Stock $50,000 40% 25% Assume that any money not invested does not earn interest. a) Draw a decision tree for this scenario-making your consequence your cash in hand at the end of one year in thousands of dollars. b) Solve this decision tree using EMV. What is the optimal decision strategy? c) If we assume that an investor's utility function is given by U(x) = Vx, again with x in thousands of dollars, what is the optimal decision and what is its expected utility (round your utilities to 2 decimal places when calculating the utilities)? Based on the utility function, is the investor risk averse, riskseeking, or risk neutral? Does the optimal investment choice make sense given that risk attitude? d) If we assume that an investor's utility function is given by U(x) = x2, again with x in thousands of dollars, what is the optimal decision and what is its expected utility? Based on the utility function, is the investor risk averse, risk seeking, or risk neutral? Does the optimal investment choice make sense given that risk attitude? You are considering three investment options (and, of course, you could choose not to invest). You have $50,000 to invest and for your consequence you will consider the cash (in thousands of dollars) that remains one year after you make your investment. The investment amounts for each of the options and the potential returns are as follows: Investment Option Investment Amount Probability 18% 60% Large Cap Stock $50,000 Investment Outcome Excellent Average Poor Excellent Average Poor Excellent Average Poor 33% 45% Value of Investment after one year $53,000 $52,000 $45,500 $56,000 $50,000 $44,500 $60,000 $55,000 $35,000 Mid Cap Stock $50,000 Small Cap Stock $50,000 40% 25% Assume that any money not invested does not earn interest. a) Draw a decision tree for this scenario-making your consequence your cash in hand at the end of one year in thousands of dollars. b) Solve this decision tree using EMV. What is the optimal decision strategy? c) If we assume that an investor's utility function is given by U(x) = Vx, again with x in thousands of dollars, what is the optimal decision and what is its expected utility (round your utilities to 2 decimal places when calculating the utilities)? Based on the utility function, is the investor risk averse, riskseeking, or risk neutral? Does the optimal investment choice make sense given that risk attitude? d) If we assume that an investor's utility function is given by U(x) = x2, again with x in thousands of dollars, what is the optimal decision and what is its expected utility? Based on the utility function, is the investor risk averse, risk seeking, or risk neutral? Does the optimal investment choice make sense given that risk attitude