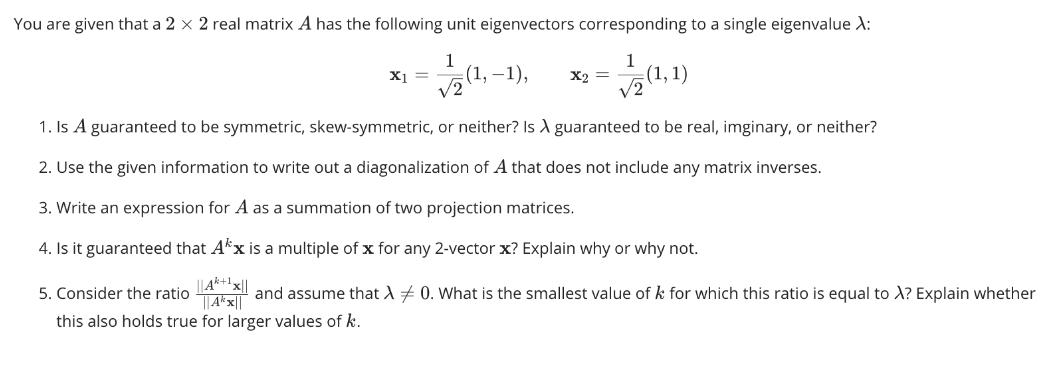
You are given that a 2 x 2 real matrix A has the following unit eigenvectors corresponding to a single eigenvalue X: 1 X2 = (1,1) 2 1 (1, -1), 2 1. Is A guaranteed to be symmetric, skew-symmetric, or neither? Is A guaranteed to be real, imginary, or neither? 2. Use the given information to write out a diagonalization of A that does not include any matrix inverses. 3. Write an expression for A as a summation of two projection matrices. 4. Is it guaranteed that Ax is a multiple of x for any 2-vector x? Explain why or why not. X1 = 5. Consider the ratio and assume that 0. What is the smallest value of k for which this ratio is equal to X? Explain whether ||Akx|| this also holds true for larger values of k.