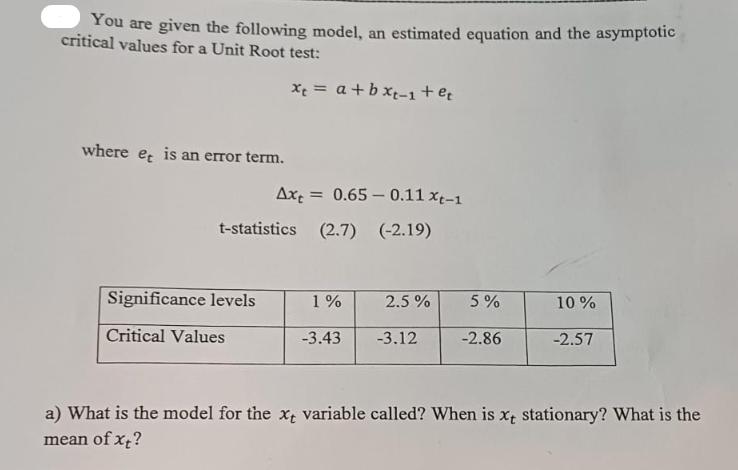
You are given the following model, an estimated equation and the asymptotic critical values for a Unit Root test: x = a +bxt-1 + et where e, is an error term. Axt = 0.65 -0.11 Xt-1 t-statistics (2.7) (-2.19) Significance levels Critical Values 1% 2.5% -3.43 -3.12 5% -2.86 10% -2.57 a) What is the model for the x variable called? When is xt stationary? What is the mean of xt? b) Calculate the estimated sample correlation coefficient of xt? What is the difference between the sample correlation coefficient and the population correlation coefficient? c) State your Null and Alternative Hypotheses for a Unit Root test of the xt variable? Why is the Rejection Region on the negative side in this test? What is the test statistic (t statistic) distributed like under the Null Hypothesis? Test formally whether xt is stationary or not? What is the other name for Unit Root testing? d) What does the time path of x, look like if the Alternative Hypothesis is correct? What is the model for x called if the Null Hypothesis is correct? Write down this model? e) What do the Autocorrelation Functions of this series look like when the Null Hypothesis and the Alternative Hypothesis are correct (separately in each case)? How would you change your estimated equation above, to conduct the Unit Root test if the error terms are found to be correlated? Will the critical values and the rejection rules change or not? What is the name of this Unit Root test now? (YOU DON'T HAVE TO WRITE AN EQUATION, JUST ANSWER VERBALLY)