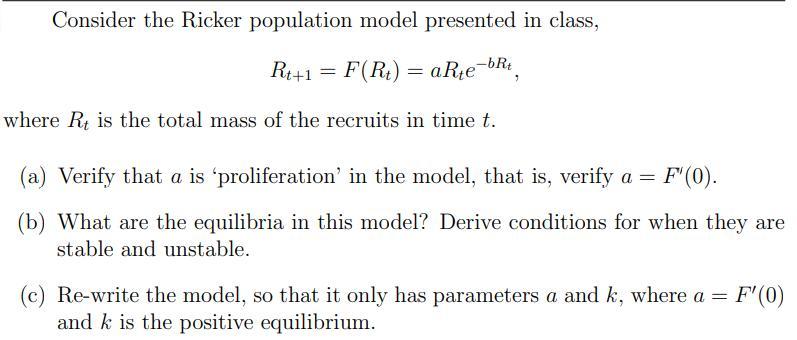
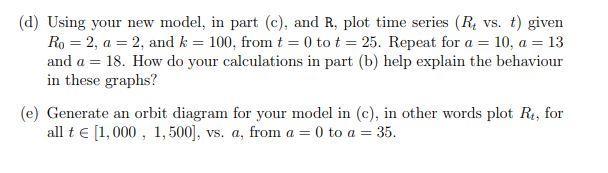
Consider the Ricker population model presented in class, Rt+1 = F(Rt) = aRte aRte-bRt where R is the total mass of the recruits in time t. (a) Verify that a is 'proliferation' in the model, that is, verify a = F'(0). (b) What are the equilibria in this model? Derive conditions for when they are stable and unstable. F'(0) (c) Re-write the model, so that it only has parameters a and k, where a = and k is the positive equilibrium. (d) Using your new model, in part (c), and R. plot time series (R, vs. t) given Ro = 2, a = 2, and k = 100, from t = 0 to t = 25. Repeat for a = 10, a = 13 and a = 18. How do your calculations in part (b) help explain the behaviour in these graphs? (e) Generate an orbit diagram for your model in (c), in other words plot R, for all t [1,000, 1, 500], vs. a, from a = 0 to a = 35.