zoom in if u cant see

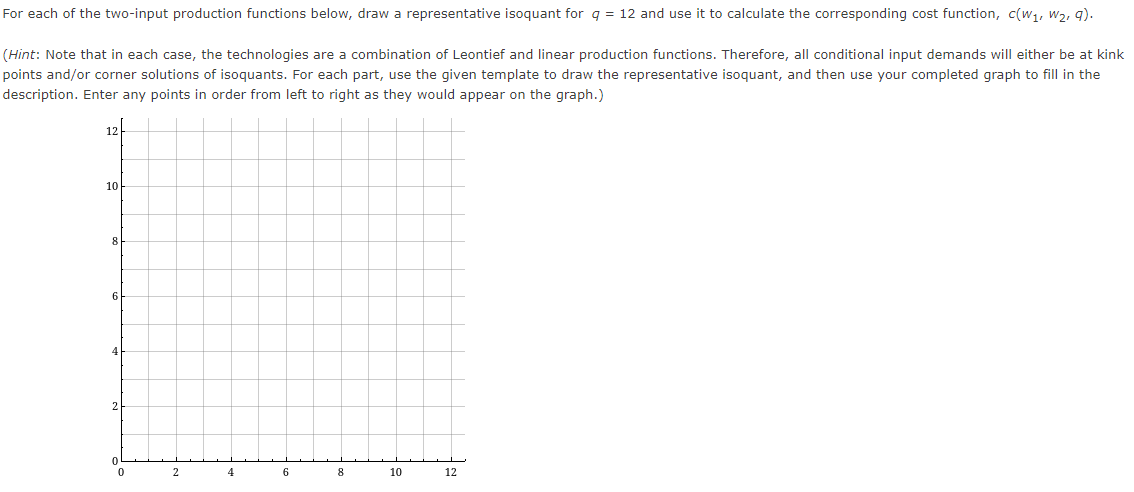
For each of the two-input production functions below, draw a representative isoquant for 9 = 12 and use it to calculate the corresponding cost function, c(W1, W2,9). (Hint: Note that in each case, the technologies are a combination of Leontief and linear production functions. Therefore, all conditional input demands will either be at kink points and/or corner solutions of isoquants. For each part, use the given template to draw the representative isoquant, and then use your completed graph to fill in the description. Enter any points in order from left to right as they would appear on the graph.) 10 8 6 4 0 0 2 4 6 8 10 12 (b) q = mi min{2x2, 2(x2 + x2), 2x2} When W1/W2 > 1, the input bundle minimizes the cost of production and costs Similarly, when W1/W2 S 1, the input bundle minimizes the cost of production and costs . Therefore, the cost function is as follows. O (W1, W2, 9) = 9 min (W1, W2, 9) = 9 min{wz + zwar zwa + wz} min{{w} + {wz. Zwy + Zw2} - min{w2 + uz, qwz + wa} {fw2 + w, w2 + wz} O cw1, W2, 9) = 9 min O c(W1, W2, 9) = 9 min W2 O (W1, W2, 9) = q. min For each of the two-input production functions below, draw a representative isoquant for 9 = 12 and use it to calculate the corresponding cost function, c(W1, W2,9). (Hint: Note that in each case, the technologies are a combination of Leontief and linear production functions. Therefore, all conditional input demands will either be at kink points and/or corner solutions of isoquants. For each part, use the given template to draw the representative isoquant, and then use your completed graph to fill in the description. Enter any points in order from left to right as they would appear on the graph.) 10 8 6 4 0 0 2 4 6 8 10 12 (b) q = mi min{2x2, 2(x2 + x2), 2x2} When W1/W2 > 1, the input bundle minimizes the cost of production and costs Similarly, when W1/W2 S 1, the input bundle minimizes the cost of production and costs . Therefore, the cost function is as follows. O (W1, W2, 9) = 9 min (W1, W2, 9) = 9 min{wz + zwar zwa + wz} min{{w} + {wz. Zwy + Zw2} - min{w2 + uz, qwz + wa} {fw2 + w, w2 + wz} O cw1, W2, 9) = 9 min O c(W1, W2, 9) = 9 min W2 O (W1, W2, 9) = q. min