Consider a system represented by a first-order ordinary differential equation: (a) Show first that for a function
Question:
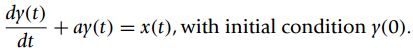
(a) Show first that for a function f(t)
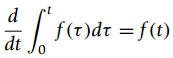
using the definition of the derivative.
(b) Apply the above result to show that the solution of the first-order ordinary differential equation with initial condition y(0) given above is
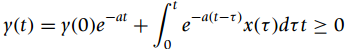
(c) Another way to show the above expression is the solution of the ordinary differential equation is to multiply both sides of the ordinary differential equation by eat
i. show that the left term is d(eat y(t))/dt,
ii. integrate the two terms to obtain the solution.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





