For couplings between spins, we can construct four independent Hermitian coupling matrices, Show explicitly, by calculating the
Question:
For couplings between spins, we can construct four independent Hermitian coupling matrices,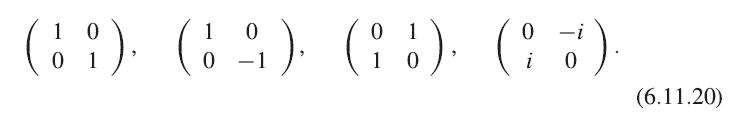
Show explicitly, by calculating the characters for the C2 and C4 rotations, that one of these matrices can act as the basis of a D+0 representation of the full rotation symmetry group, and the other three can act as the basis functions for a D+1 representation, that is, they transform as Lx, Ly, Lz. The rotation matrix acting on a spinor, for a rotation of angle θ around an axis n̂, is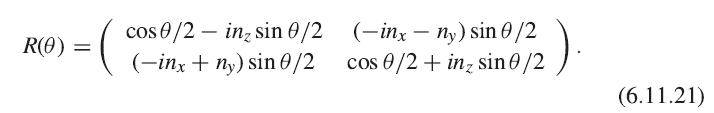
By the method of invariants, we can then multiply each by the magnetic field component that transforms the same way, to get the Hamiltonian for the effect of magnetic field on a particle with spin. Write down this full Hamiltonian. Note that spin, angular momentum, and magnetic field are all even under an inversion transformation I.
Step by Step Answer:
The characters of the full rotation group from Table 613 in the book are E C6 C4 C3 C2 D D C 1 3 A C ...View the full answer
