Show that (4.2.13) is equal to (4.2.14) by substituting into (4.2.14) the definitions of a k and
Question:
Show that (4.2.13) is equal to (4.2.14) by substituting into (4.2.14) the definitions of ak and a†k. You will need to use the definitions of xk and pk in terms of xn and pn, and the relation [xn, pn′ ] = ih̄δn,n′.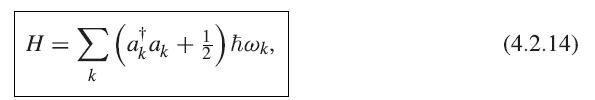
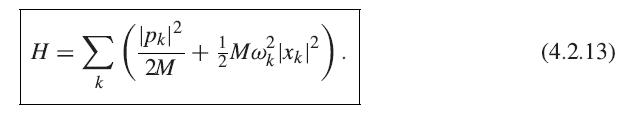
Transcribed Image Text:
= Σ (atak + 2 ) ha hok, k H = (4.2.14)
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 100% (4 reviews)
and From Eq 414 and Eq 423 we have Mwk k Pk 1 N 1 V...View the full answer

Answered By

Utsab mitra
I have the expertise to deliver these subjects to college and higher-level students. The services would involve only solving assignments, homework help, and others.
I have experience in delivering these subjects for the last 6 years on a freelancing basis in different companies around the globe. I am CMA certified and CGMA UK. I have professional experience of 18 years in the industry involved in the manufacturing company and IT implementation experience of over 12 years.
I have delivered this help to students effortlessly, which is essential to give the students a good grade in their studies.
3.50+
2+ Reviews
10+ Question Solved
Related Book For 

Question Posted:
Students also viewed these Sciences questions
-
The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...
-
The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-4. Ivan and Irene paid the following in 2012 (all by check or can otherwise be...
-
A compare-exchange operation on two array elements A[i] and A[j], where i < j, has the form COMPARE-EXCHANGE (A, i, j) 1 If A[i] > A[j] 2 exchange A[i] with A[j] After the compare-exchange operation,...
-
Greenback Sporting, a retailer of outdoor wear and sporting equipment, is investigating an opportunity to purchase Active Lifestyle Inc. An acquisition is expected to lower overhead costs, improve...
-
During 2014, Cumpuincs first year of operations, the company reports pretax financial income at $231,000. Cumpuincs enacted tax rate is 40% for 2014 and 2015 and 30% for all later years. Cumpuinc...
-
On January 1, 1998, Chain Corporation issued \($5\) million of 7% coupon bonds at par. The bonds mature in 20 years and pay interest semiannually on June 30 and December 31 of each year. On December...
-
Special interest groups operate in most countries. They tend to have distinctive agendas. What is their role in the political and legal system? LO.1
-
Breakeven point, what-if analysis Premier Products, Inc., is considering replacing its existing machine with a new and faster machine that will produce a more reliable product and will turn around...
-
Stacey Lang, 48 years old, has lived in George for the past 30 years. Stacey was relocated in the current year to Johannesburg after accepting an executive director position at ABCs Head Office....
-
Convert (4.9.10) into an equation for the radiant energy per unit wavelength for a blackbody radiation source. From this equation, determine the peak emission wavelength, that is, the wavelength at...
-
Prove the statement given in this section that the time evolution operator is unitary, under the assumption that the norm of the wave function t | t = 1 at all times. S(t, to) = e(i/h) Vint(t)dt'
-
Find the acute angle for each of the given trigonometric functions. Round off results according to Table 4.1. csc = 1.002 Data from Table 4.1 Angles and Accuracy of Trigonometric Functions...
-
Watch the clip from HBO's Chernobyl and respond to the questions below using complete sentences....
-
1) Every organization has a unique culture. As you move forward with a travel or per diem position, what steps will you take to learn about your assignment organization's culture? 2)Flexibility and...
-
See the right figure of the lifting and transporting equipment. During operation, while a mass of 1.5 x 104 kg was descending at a constant speed v = 15 m/min, the machine experienced a breakdown,...
-
PROBLEM 3 BABEY Company makes three products with the following characteristics: Product V jk jin Selling price per unit 10 15 20 Variable cost per unit 6 10 10 Machine hours per unit 2 4 10 The...
-
Diversified Semiconductors sells perishable electronic components. Some must be shipped and stored in reusable protective containers. Customers pay a deposit for each container received. The deposit...
-
Imagine that Serious Materials has hired you as an HR consultant for the Chicago window factory. Suggest how the company can build on its initial goodwill with workers to create positive labor...
-
In the busy port of Chennai, India, the number of containers loaded onto ships during a 15-week period is as follows: 1. Develop a linear trend equation to forecast container loadings. 2. Using the...
-
Give an example of two systems that are in equilibrium with respect to only one of two state variables.
-
Aerobic cells metabolize glucose in the respiratory system. This reaction proceeds according to the overall reaction 6O 2 (g) + C 6 H 12 O 6 (s) 6CO 2 (g) + 6H 2 O(l) Calculate the volume of oxygen...
-
Using acetylene as your only source of carbon atoms, outline a synthesis for 3-hexyne.
-
Which of the following concerning short-term financing methods is NOT CORRECT? Short-term bank loans typically do not require assets as collateral. Firms generally have little control over the level...
-
Kingbird Corporation is preparing its December 31, 2017, balance sheet. The following items may be reported as either a current or long-term liability. 1. On December 15, 2017, Kingbird declared a...
-
BE13.2 (LO 1), AP An inexperienced accountant for Silva Corporation showed the following in the income statement: net income \$337,500 and unrealized gain on availablefor-sale securities (before...

Study smarter with the SolutionInn App


