(a) Show that the variance of the Poisson distribution whose probability function is shown in Table 3.1...
Question:
(a) Show that the variance of the Poisson distribution whose probability function is shown in Table 3.1 is \(\lambda\), the same as the mean of the distribution.
(b) Show that the mean of the \(\mathrm{U}(0,1)\) distribution is \(1 / 2\) and the variance is \(1 / 12\).
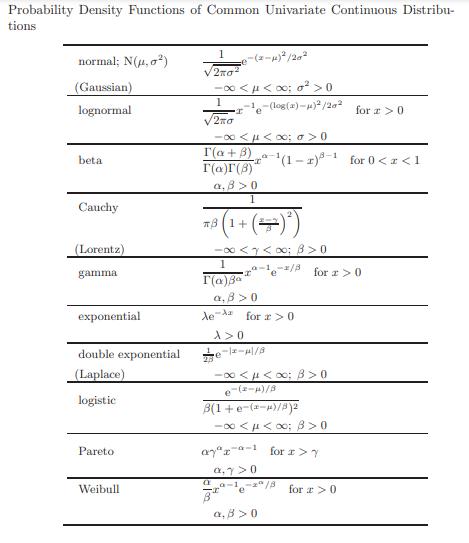
(c) Show that the mean and variance of the gamma distribution with the PDF shown in Table 3.2 are \(\alpha \beta\) and \(\alpha \beta^{2}\).
(d) Use the PDF of the gamma distribution to evaluate the integral
\[ \int_{0}^{\infty} \frac{1}{1728} \lambda^{13} \mathrm{e}^{-5 \lambda} \mathrm{d} \lambda \]
(e) Let \(X\) have the triangular distribution. The triangular distribution has PDF
\[ f_{X}(x)= \begin{cases}1-|x| & \text { for }-1 Show that \(\mathrm{E}(X)=0\) and \(\mathrm{V}(X)=\frac{1}{6}\). Table 3.1: Table 3.2: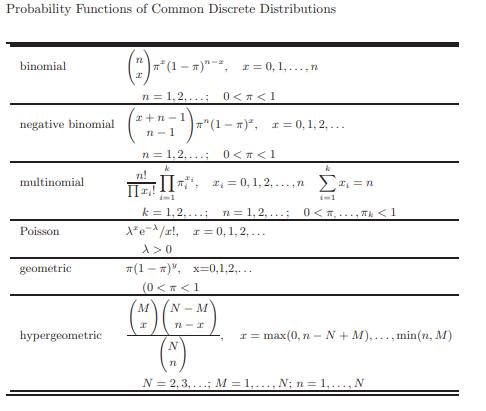
Step by Step Answer:






