Refer to the IHS Journal of Hydraulic Engineering (September 2012) study of the repair and replacement of water pipes, Exercise 11.37. Recall that a team of civil engineers used regression analysis to model y = the ratio of repair to replacement cost of commercial pipe as a function of x = the diameter (in millimeters) of the pipe. Using data for a sample of 13 different pipe sizes (see below) you fit the quadratic model, E(y) = β₀ + β₁x₁ + β₂x² . Is there a high level of multicollinearity in the independent variables? If so, propose an alternative model that does not suffer from the same high level of multicollinearity. Fit the model to the data and interpret the results.

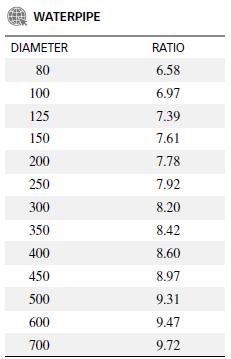
Data from Exercise 11.37

Refer to the IHS Journal of Hydraulic Engineering (September, 2012) study of the repair and replacement of water pipes, Exercise 10.8. Recall that a team of civil engineers used regression analysis to model y = the ratio of repair to replacement cost of commercial pipe as a function of x= the diameter (in millimeters) of the pipe. Data for a sample of 13 different pipe sizes are reproduced in the accompanying table. In Exercise 10.8, you fit a straight-line model to the data.

Transcribed Image Text:

WATERPIPE DIAMETER RATIO 80 6.58 100 6.97 125 7.39 150 7.61 200 7.78 250 7.92 300 8.20 350 8.42 400 8.60 450 8.97 500 9.31 600 9.47 700 9.72