The daily production of electricity generated by a coal-fired power plant operating in the midwest can be represented as

\(Y_{i}=\beta_{1} l_{i}^{\beta_{2}} m_{i}^{\beta_{3}} e^{\varepsilon_{i}}\)

where

\(Y_{i}=\) quantity of electricity produced on day \(i\), measured in megawatts;

\(l_{i}=\) quantity of labor input used on day \(i\), measured in 100 's of hours;

\(m_{i}=\) units of fossil fuel input on day \(i_{\text {; and }}\)

\(\varepsilon_{i} \sim N\left(0, \sigma^{2}ight)\), the \(\varepsilon_{i}^{\prime}\) s being iid.

One hundred days of observations on the levels of inputs used and the quantity of electricity generated were collected. The following information is provided to you by the utility company, where \(\mathbf{y}_{*}=\ln (\mathbf{y})\), and \(\mathbf{x} *\) is a matrix consisting of a vector of 1's followed by column vectors corresponding to the natural logarithms of labor and fuel levels.

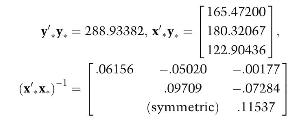
(a) Transform the production function into a form in which parameters can be estimated using the least squares estimator. Estimate the parameters of the transformed model.

(b) Is the estimator you used in part

(a) to estimate \(\mathbf{q}(\boldsymbol{\beta})\) (1) unbiased, (2) asymptotically unbiased, (3) BLUE, (4) MVUE, (5) consistent, and/or (6) normally distributed?

(c) Is the estimator you used to estimate \(\sigma^{2}\) (1) unbiased, (2) asymptotically unbiased (3) BLUE, (4) MVUE, (5) consistent, and/or (6) gamma-distributed?

(d) Define the MVUE for the degree of homogeneity of the production function (i.e., define the MVUE for \(q_{*}(\boldsymbol{\beta})=\beta_{2}+\beta_{3}\). Estimate the degree of homogeneity of the production function using the MVUE. Is the MVUE a consistent estimator of the degree of homogeneity? Is the MVUE normally distributed?

(e) Define an MVUE estimator for the covariance matrix of the least-squares estimator. Estimate the covariance matrix of the least-squares estimator.

Transcribed Image Text:

y'.y. = 288.93382, x'.y. (X'.x.)- = .06156 165.47200 180.32067 122.90436 -.05020 -.00177 .09709 -.07284 (symmetric) 11537