Consider the following differential equation: Assume a solution of the form: (tilde{u}(x)=c_{1} x+c_{2} x^{2}). Calculate the unknown

Question:

Consider the following differential equation:

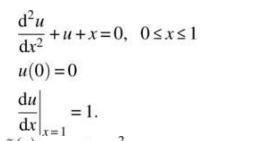
image text in transcribed

Assume a solution of the form: $\tilde{u}(x)=c_{1} x+c_{2} x^{2}$. Calculate the unknown coefficients using the Galerkin method. Compare $u(x)$ and $d u(x) / d x$ with the exact solution: $u(x)=3.7 \sin x-x$ by plotting the solution.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Introduction To Finite Element Analysis And Design

ISBN: 9781119078722

2nd Edition

Authors: Nam H. Kim, Bhavani V. Sankar, Ashok V. Kumar

Question Posted: Mar 16, 2024 12:21 PM

See More Questions

Q1. You have identified a market opportunity for home media players that would cater for older members of the population. Many older people have difficulty in understanding the operating principles...
If F is a function of V and T, prove that -T), -v), F H = FT), -v() F G = F V T
answer all questions as instructed below. attend all questions. 4 Computer Vision (a) Explain why such a tiny number of 2D Gabor wavelets as shown in this sequence are so efficient at representing...
1. Suppose India follows China and accelerates its industrialization. For India, this will: A. Decrease imports and raise net exports B. Increase imports and lower net exports C. Increase imports and...
Consider the Spearman rank correlation coefficient rs for data pairs (x, y). What is the monotone relationship, if any, between x and y implied by a value of (a) rs = 0? (b) rs close to 1? (c) rs...
Can process costing be used for a service organization? Explain. Describe how process costing can be used for JIT manufacturing firms. LO1
What do you recommend that Cardone Industries do to compete more successfully with auto parts rebuilders in China? LO.1
Presented below are the financial statements of Rajesh Company Ltd. RAJESH COMPANY LTD. Income Statement For the Year Ended December 31, 2017 Sales...
W. W. Phillips Company produced 4,000 leather recliners during the year. These recliners sell for $400 each. Phillips had 500 recliners in finished goods inventory at the beginning of the year. At...
The uniaxial bar shown in figure 2.21 is of uniform cross sectional area $A$ and length $L$. It is clamped at the left end and subjected to a concentrated force $F$ at the right end as shown....
Solve the differential equation given in eq. (2.1) for $p(x)=1$ using the Galerkin finite element method. Assume that the domain $0 \leq x \leq 1$ is divided into two equallength elements....
Liberty Ventures Inc. reported $209,840 profit in 2023 and declared preferred dividends of $34,400. The following changes in common shares outstanding occurred during the year: Calculate the...
Begin by locating a scholarly article regarding the effects of increases on minimum wages and discuss its findings. Does a minimum wage increase lead to reductions in employment, or is the overall...
Pick an organization (or you can use an example that has professional relevance), and use the DAC framework to accomplish the following objectives. Provide background and a problem statement of the...
Woodruff Company is currently producing a snowmobile that uses five specialized parts. Engineering has proposed replacing these specialized parts with commodity parts, which will cost less and can be...
What was Newell's organizational culture like before acquiring Rubbermaid? What was the quadrant? Is the perspective short or long term, inside or outside focused, interested in people, process, or...
(7.47 of Koretsky 2nd edition) A binary mixture of species 1 and 2 can be described by the following equation of state: P = a 0 VoT The pure species coefficients are given by: amix y1a1a2Y2 with the...
Refer to Exercise 12.11. Display the Y and X matrices for the following two prediction models: a. = 0 + 1 AGE + 2 Weight b. = 0 + 1 AGE + 2 Weight + 3 AGE2 + 4 Weight2 + 5 AGE? Weight In exercise lic...
Show that, given a maximum flow in a network with m edges, a minimum cut of N can be computed in O(m) time.
Explain the need for a relational database for PCs. What improvements has it seen over the years?
Each team member should design an appropriate input display for Maverick Transport using either a CASE tool, a drawing tool such as Microsoft Visio, or paper and pencil. Share your results with your...
The Carol, Tracy, & Leslie Realty Corporation specializes in locating homes for prospective buyers. Home information is stored in a database and is to be shown on an inquiry display screen. Design a...
Which of the following statements regarding traditional cost accounting systems is false? a. Products are often over or under cost in traditional cost accounting systems. b. Most traditional cost...
Bart is a college student. Since his plan is to get a job immediately after graduation, he determines that he will need about $250,000 in life insurance to provide for his future wife and children...
Reporting Financial Statement Effects of Bond Transactions (please show me how you got the answers) Lundholm, Inc., which reports financial statements each December 31, is authorized to issue...

Consider the following differential equation: Assume a solution of the form: (tilde{u}(x)=c_{1} x+c_{2} x^{2}). Calculate the unknown

Question:

du +u+x=0, 0x1 dr u(0)=0 du = 1. dr x=1

Step by Step Answer:

Introduction To Finite Element Analysis And Design

Students also viewed these Computer science questions