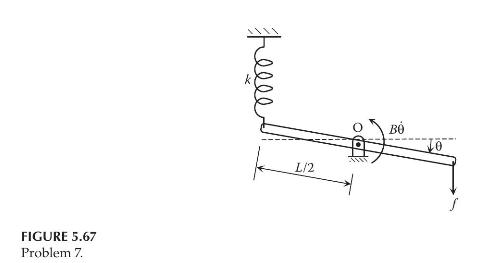
The system shown in Figure 5.67 consists of a uniform rod of mass \(M\) and length \(L\) and a translational spring of stiffness \(k\) at the rod's left tip. The friction at the joint \(\mathrm{O}\) is modeled as a damper with coefficient of torsional viscous damping \(B\). The input is the force \(f\) and the output is the angle \(\theta\). The position \(\theta=0\) corresponds to the static equilibrium position when \(f=0\).

a. Draw the necessary free-body diagram and derive the differential equation of motion for small angles \(\theta\).

b. Using the linearized differential equation obtained in Part (a), determine the transfer function \(\Theta(s) / F(s)\). Assume that the initial conditions are \(\theta(0)=0\) and \(\dot{\theta}(0)=0\).

c. Using the differential equation obtained in Part (a), determine the state-space representation.

Transcribed Image Text:

FIGURE 5.67 Problem 7. heeee L/2 BO 0