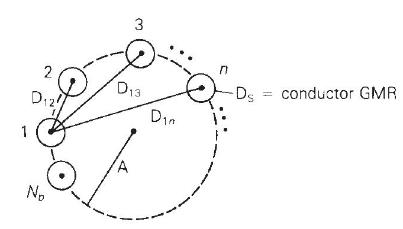
A bundle configuration for UHV lines (above \(1000 \mathrm{kV}\) ) has identical conductors equally spaced around a circle, as shown in Figure 4.29. \(N_{b}\) is the number of conductors in the bundle, \(A\) is the circle radius, and \(D_{S}\) is the conductor GMR. Using the distance \(\mathrm{D}_{1 n}\) between conductors 1 and \(n\) given by \(\mathrm{D}_{1 n}=2 \mathrm{~A} \sin \left[(n-1) \pi / N_{b}ight]\) for \(n=1,2, \ldots, N_{b}\), and the following trigonometric identity:

\(\left[2 \sin \left(\pi / N_{b}ight)ight]\left[2 \sin \left(2 \pi / N_{b}ight)ight]\left[2 \sin \left(3 \pi / N_{b}ight)ight] \cdots\left[2 \sin \left\{\left(N_{b}-1ight) \pi / N_{b}ight\}ight]=N_{b}\)

show that the bundle GMR, denoted \(\mathrm{D}_{\mathrm{SL}}\), is

\(\mathrm{D}_{\mathrm{SL}}=\left[N_{b} \mathrm{D}_{\mathrm{S}} \mathrm{A}^{\left(N_{b}-1ight)}ight]^{\left(1 / N_{b}ight)}\)

Also show that the above formula agrees with (4.6.19) through (4.6.21) for EHV lines with \(N_{b}=2,3\), and 4 .

1 2 D2 No 3 D13 Din n Ds conductor GMR