Calculation problem: Standard error and confidence intervals of the slope. How uncertain is our estimate of slope?

Question:

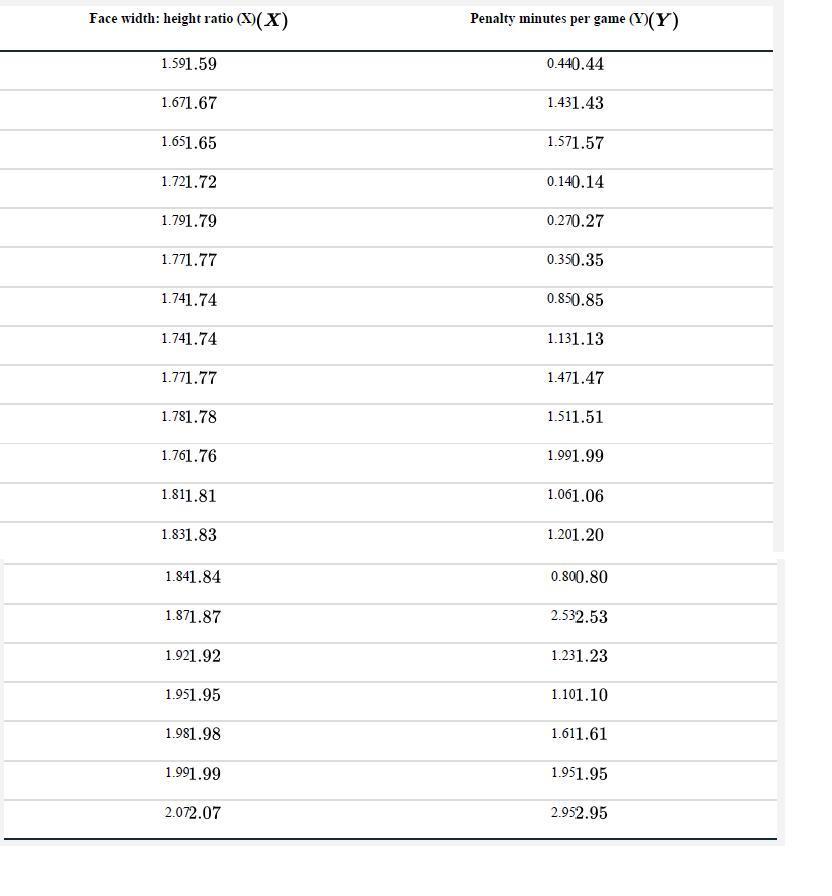
Calculation problem: Standard error and confidence intervals of the slope. How uncertain is our estimate of slope? Using the face ratio and hockey aggressive penalty data from Practice Problem 1, calculate the standard error and confidence interval of the slope of the linear regression.

a. Calculate the total sum of squares for the response variable, penalty minutes.

b. Calculate the residual mean square MS_residual , using the total sum of square for Y , the sum of products, and the slope b.

c. With the sum of squares for X and MSresidual , calculate the standard error of b.

d. How many degrees of freedom does this analysis of the slope have?

e. Find the two-tailed critical t-value for a 95% confidence interval (α=0.05) for the appropriate df.

f. Calculate the confidence interval of the population slope, β.

Data from Problem 1

Calculation problem: Regression lines. Men’s faces have higher width-to-height ratios than women’s, on average. This turns out to reflect a difference in testosterone expression during puberty.

Testosterone is also known to predict aggressive behavior. Does face shape predict aggression? To test this, Carré and McCormick (2008) compared the face width-to-height ratio of 21 university hockey players with the average number of penalty minutes awarded per game for aggressive infractions like fighting or cross-checking. Their data are below along with some partial calculations. We will calculate the equation for the line that best predicts penalty minutes from face width-to-height ratio.

Face width: height ratio (X)(X) 1.591.59 1.671.67 1.651.65 1.721.72 1.791.79 1.771.77 1.741.74 1.741.74