Show that the free energy of an ideal monatomic gas of N 3 particles in a volume
Question:
Show that the free energy of an ideal monatomic gas of N3 particles in a volume V can be written as F =−kBN3T (1 − ln(N3λ3/V))
, where 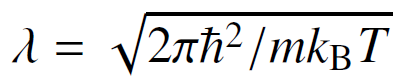
is a thermal length scale. Show that a two-dimensional ideal gas of N2 particles moving in a bounded area A has free energy 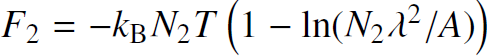
Transcribed Image Text:
1%3D V2лћ?/mkвТ F2 = -KBN2T (1 – In(N2x²/A))
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 55% (9 reviews)
First consider three dimensions From eq And Z ideal V 3N 3 2 N 3 For a free mona...View the full answer

Answered By

Utsab mitra
I have the expertise to deliver these subjects to college and higher-level students. The services would involve only solving assignments, homework help, and others.
I have experience in delivering these subjects for the last 6 years on a freelancing basis in different companies around the globe. I am CMA certified and CGMA UK. I have professional experience of 18 years in the industry involved in the manufacturing company and IT implementation experience of over 12 years.
I have delivered this help to students effortlessly, which is essential to give the students a good grade in their studies.
3.50+
2+ Reviews
10+ Question Solved
Related Book For 

Question Posted:
Students also viewed these Physics questions
-
Show that the free energy change for the succinate dehydrogenase reaction catalyzed by Complex II is insufficient to drive ATP synthesis under standard conditions.
-
The atoms of an ideal monatomic gas of N particles confined in a box of volume V can be adsorbed onto the surface of the box (surface area A), where they are bound to the surface with binding energy...
-
The free energy of formation of one mole of compound refers to a particular chemical equation. For each of the following, write that equation. a. KBr(s) b. CH3Cl(l) c. H2S(g) d. AsH3(g)
-
A red laser ( lambda r = 6 3 3 nm ) is shone through a narrow slit located 2 m from a screen. When the light source is replaced by a blue laser ( b = 4 8 8 nm ) the position of the first minimum of...
-
Two identical cars, one on the moon and one on the earth, have the same speed and are rounding banked turns that have the same radius r. There are two forces acting on each car, its weight mg and the...
-
In Problem 1-8, name the conic (horizontal ellipse, vertical hyperbola, and so on) corresponding the given equation. 1. x2/9 + y2/4 = 1 2. x2/9 - y2/4 = 1 3. - x2/9 + y2/4 = 1 4. -x2/9 + y2/4 = -1 5....
-
(b) Analyse the response variable porosity_final by analysis of variance and by mixed modelling. The term porosity_initial can be added to the mixed model.
-
PAR AND NO-PAR, COMMON AND PREFERRED STOCK Valdez Company had the following stock transactions during the year: (a) Issued 24,000 shares of $1 par common stock for $26,000 cash. (b) Issued 20,000...
-
17. You bought a home in cash for $350,000. Ten years later you sold the house for $475,000. What is the annual rate of return with monthly compounding on the investment? Please enter the percentage,...
-
Higgins, Inc., has sales of $517,400, costs of $296,300, depreciation expense of $42,300, interest expense of $20,400, and a tax rate of 21 percent. What is the net income for the firm? Suppose the...
-
Show that when a system comes into thermal equilibrium while in contact with a heat reservoir at temperature T, its free energy (Helmholtz, if V is held fixed; Gibbs if p is held fixed) is minimized.
-
The universe is filled with electromagnetic radiation left over from the Big Bang. This radiation has a characteristic temperature of 2.7K. At what wavelength does the power spectrum of this...
-
What are direct costs? Opportunity costs? Why should we not include both when constructing our cost matrix?
-
On January 1, 20X1, Popular Creek Corporation organized SunTime Company as a subsidiary in Switzerland with an initial investment cost of Swiss francs (SFr) 76,000. SunTime's December 31, 20X1, trial...
-
In November 2 0 2 4 , Lily informed you that she needs additional cash flow to meet her personal debt obligations. Lily does not want to sell more stock than she needs to because she wants...
-
Bennett limited provides mobile library services to the community of longbourn. bennett has preliminary operating results for the first year and the company found that net income is different from...
-
What is printed when the value of x is 34? if (x < 32 ) { if (x22) { } System.out.println("Blue"); else if (x <10) { } System.out.println("Red"); else if (x > 25 ) { System.out.println("Yellow"); } }...
-
Heidi expresses concern in the video that when employees are also friends, holding them accountable for performance "doesn't come as naturally" to her. She asks you, "Does my focus on relationship...
-
After a job interview, you should send a follow-up message a. Within two days after the interview. b. Only if you think you got the job. c. That follows the AIDA organizational model. d. That does...
-
Calculate the change in entropy when 100 kJ of energy is transferred reversibly and isothermally as heat to a large block of copper at (i) 0 C, (ii) 50 C.
-
As an intern with an engineering firm, you are asked to measure the moment of inertia of a large wheel, for rotation about an axis through its center. Since you were a good physics student, you know...
-
A meter stick with a mass of 0.180 kg is pivoted about one end so it can rotate without friction about a horizontal axis. The meter stick is held in a horizontal position and released. As it swings...
-
The pulley in Fig. P9.84 has radius 0.160 m and moment of inertia 0.560 kg m 2 . The rope does not slip on the pulley rim. Use energy methods to calculate the speed of the 4.00-kg block just before...
-
If the auditor believes that the financial statements prepared on the basis of the entity's income tax are not adequately titled, the auditor should : A)Issue a resignation of opinion. B)Explain the...
-
initial stock offering to the public. This REIT specializes in the acquisition and management of warehouses. Your firm, Blue Street Advisors, is an investment management company that is considering...
-
Question 3 You have been hired to run a pension fund for Mackay Inc, a small manufacturing firm. The firm currently has Gh5 million in the fund and expects to have cash inflows of $2 million a year...

Study smarter with the SolutionInn App


