Consider a miner trapped in a room that contains three doors. Door 1 leads him to freedom after two days of travel; door 2 returns him to his room after a four-day journey; and door 3 returns him to his room after a six-day journey.

Suppose at all times he is equally likely to choose any of the three doors, and let T denote the time it takes the miner to become free.

(a) Define a sequence of independent and identically distributed random variables X1,X2 . . . and a stopping time N such that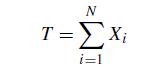

Note: You may have to imagine that the miner continues to randomly choose doors even after he reaches safety.

(b) Use Wald’s equation to find E[T ].

(c) Compute E /N i=1Xi |N = n 0 and note that it is not equal to E[n i=1Xi ].

(d) Use part

(c) for a second derivation of E[T ].

Transcribed Image Text:

N Xi T=EX i=1