This cumulative review problem uses material from Chapters 3, 5, and 10. Recall that the Poisson distribution deals with rare events. Death from the kick of a horse is a rare event, even in the Prussian army. The following data are a classic example of a Poisson application to rare events.

A reproduction of the original data can be found in C. P. Winsor, Human Biology, Vol. 19, pp. 154–161. The data represent the number of deaths from the kick of a horse per‑army corps per year for 10 Prussian army corps for 20 years (1875–1894). Let x represent the number of deaths and f the frequency of x deaths.

(a) First, we ­t the data to a Poisson distribution (see Section 5.4).

Poission distribution:

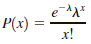
Where λ ≈ x̅ (sample mean of x values) From our study of weighted averages (see section 3.1),

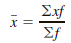
Verify that x̅ ≈ 0.61 For the category 3 or more, use 3.

(b) Now we have

1, 2, 3. p

Find P(0), P(1), P(2), and P(3 ≤ x). Round to three places after the decimal.

(c) The total number of observations is ∑f = 200.

For a given x, the expected frequency of x deaths is 200P(x). The following table gives the observed frequencies O and the expected frequencies E = 200P(x).

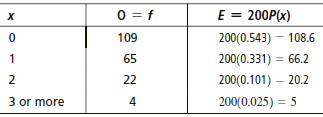
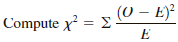
(d) State the null and alternate hypotheses for a chi-square goodness-of-­t test. Set the level of signifi­cance to be a = 0.01. Find the P-value for a goodness-of-­t test. Interpret your conclusion in the context of this application. Is there reason to believe that the Poisson distribution ­fits the raw data provided by the Prussian army? Explain.

3 or more 0 4 109 65 0.61(0.6 e-0.61 (0.61 )x for x 0. P(x) 0=f 109 65 E = 200P(x) 200(0.543) 108.6 200(0.331) 662 200(0.101) 202 2000.025)-5 0 3 or more 4 Compute X2 =