Velocity distribution for creeping flow toward a slot (Fig. 3B.7) it is desired to get the velocity
Question:
Velocity distribution for creeping flow toward a slot (Fig. 3B.7) it is desired to get the velocity distribution given for the upstream region in the previous problem. We postulate that vθ = 0, vz = 0, v, = vr (r = θ), and P = P(r, θ).
(a) Show that the equation of continuity in cylindrical coordinates gives v, = f(θ)/r, where f(θ) is a function of θ for which df/dθ = 0 at θ = 0, and f = 0 at θ = π/2.
(b) Write the r- and θ-components of the creeping flow equation of motion, and insert the expression for f(θ) from (a).
(c) Differentiate the r-component of the equation of motion with respect to θ and the θ-component with respect to r. Show that this leads to
(d) Solve this differential equation and obtain an expression for f(θ) containing three integration constants.
(e) Evaluate the integration constants by using the two boundary conditions in (a) and the fact that the total massflow rate through any cylindrical surface must equal w. This gives
(f) Next from the equations of motion in (b) obtain P(r, θ) as what is the physical meaning of P∞?
(g) Show that the total normal stress exerted on the solid surface at 0 = π/2 is
(h) Next evaluate to on the same solid surface.
(i) Show that the velocity profile obtained in Eq. 3B.8-2 is the equivalent to Eqs. 3B.7-2 and 3
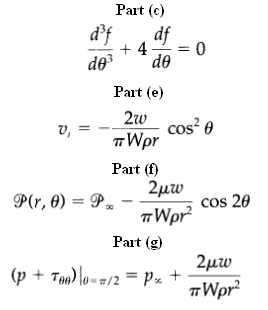
The word "distribution" has several meanings in the financial world, most of them pertaining to the payment of assets from a fund, account, or individual security to an investor or beneficiary. Retirement account distributions are among the most...
Step by Step Answer:
1 ro 0 d r Velocity distribution for creeping flow toward a slot a For the given postulates the equation of continuity gives 0 Since the flow is symme...View the full answer
