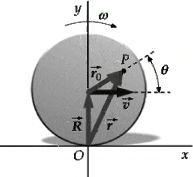
A wheel of radius R rolls without slipping at a speed V. The coordinates of the center of the wheel are X, Y. 

(a) Show that the x and y coordinates of point P in Figure are X + r₀ cos θ and R + r₀ sin θ, respectively,. 

(b) Show that the total velocity v of point P has the components v_x = V + (r₀V sin θ)/R and 

v_y = -(r₀V cos θ)/R. 

(c) Show that at the instant that X = 0, v and r are perpendicular to each other by calculating v·r.

(d) Show that v = rω, where ω = V/R is the angular velocity of the wheel. These results demonstrate that, in the case of rolling without slipping, the motion is the same as if the rolling object were instantaneously rotating about the point of contact with an angular speed w = V/R.

Transcribed Image Text:

P. ro