A discrete-time lowpass filter is to be designed by applying the impulse invariance method to a continuous-
Question:
A discrete-time lowpass filter is to be designed by applying the impulse invariance method to a continuous- time Butterworth filter having magnitude-squared function
The specifications for the discrete-time system are those of Example 7.2, i.e.,
0.89125 ≤ |H(ejω) | ≤ 1, 0 ≤ |ω| ≤ 0.2 π,
|H(ejω) | ≤ 0.17783, 0.3π ≤ |ω| ≤ π.
Assume, as in that example, that aliasing will not be a problem; i.e., design the continuous-time Butterworth filter to meet passband and stopband specifications as determined by the desired discrete-time filter.
(a) Sketch the tolerance bounds on the magnitude of the frequency response, |Hc(jΩ)|, of the continuous-time Butterworth filter such that after application of the impulse invariance method (i.e., h[n] = Tdhc(nTd)), the resulting discrete-time filter will satisfy the given design specifications. Do not assume that Td = 1 as in Example 7.2.
(b) Determine the integer order N and the quantity TdΩc such that the continuous-time Butterworth filter exactly meets the specifications determined in part (a) at the pass band edge.
(c) Note that if Td = 1, your answer in part (b) should give the values of N and Ωc obtained in Example 7.2. Use this observation to determine the system function Hc (s) for Td ≠ 1 and to argue that the system function H (z) which results from impulse invariance design with Td ≠ 1 is the came as the result for Td = 1given by Eq. (7.19).
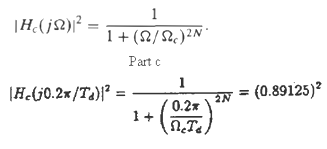
Step by Step Answer:

Discrete Time Signal Processing
ISBN: 978-0137549207
2nd Edition
Authors: Alan V. Oppenheim, Rolan W. Schafer





