A horizontal turntable rotates with angular speed . There is a clock at the center of the
Question:
A horizontal turntable rotates with angular speed ω. There is a clock at the center of the turntable and one at a distance r from the center. In an inertial reference frame, the clock at distance r is moving with speed u = rω.
(a) Show that from time dilation according to special relativity, time intervals Δt0 for the clock at rest and Δtr for the moving clock are related by
.png)
(b) In a reference frame rotating with the table, both clocks are at rest. Show that the clock at distance r experiences a pseudoforce Fr = mrω2 in this accelerated frame and that this is equivalent to a difference in gravitational potential between r and the origin of фr -ф0 = ½r2ω2. Use this potential difference in Equation 2 to show that in this frame the difference in time intervals is the same as in the inertial frame.
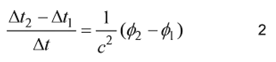
Step by Step Answer:

Fundamentals of Ethics for Scientists and Engineers
ISBN: 978-0195134889
1st Edition
Authors: Edmund G. Seebauer, Robert L. Barry





