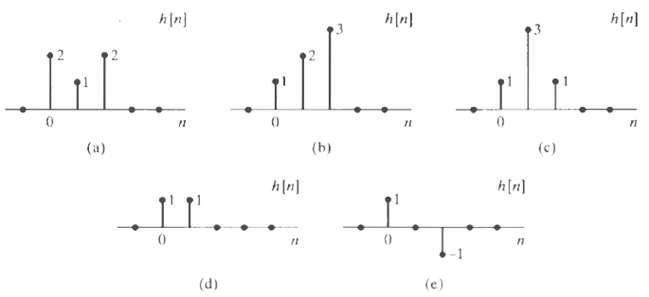
Consider the class of discrete-time filters whose frequency response has the form?

H(e^j?) = |H(e^j?)|e^?jaw,

?where |H(e^j?)| is a real and nonnegative function of ? and ? is a real constant. As discussed in Section 5.7.1, this class of filters is referred to as linear-phase filter. Consider also the class of discrete-time filters whose frequency response has the form?

H(e^j?) = A(e^j?)e^{?jaw + j?},?

Where A(e^j?) is a real function of ?, ? is a real constant, and ? is a real constant. As discussed in Section 5.7.2, filters in this class are referred to as generalized linear-phase filters. For each of the filters in Figure, determine whether it is a generalized linear-phase filter. If it is, then find A(e^j?), ? , and ?. In addition, for each filter you determine to be a generalized linear-phase filter, indicate whether it also meets the more stringent criterion for being a linear-phase filter.

h[n] h[n} (a) (b) (c) h[n] IT.. (d) (e)