Consider the simple regression yt = xt + 1 where E p[ | x] = 0 and
Question:
Consider the simple regression yt = βxt + ε1 where E p[ε | x] = 0 and E [ε2 | x ] = σ2
(a) What is the minimum mean squared error linear estimator of β? Choose e to minimize Var [β] + [E(β ?? β)]2. The answer is a function of the unknown parameters].
(b) For the estimator in part a, show that ratio of the mean squared error of β to that of the ordinary least squares estimator b is Note that τ is the square of the population analog to the ??t ratio?? for testing the hypothesis that β = 0, which is given in (4-14). How do you interpret the behavior of this ratio as τ ?? ???
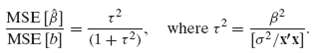
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





