In Section 10.3 we defined the time-dependent Fourier transform of the signal x[m] so that, for fixed
Question:
In Section 10.3 we defined the time-dependent Fourier transform of the signal x[m] so that, for fixed n, it is equivalent to the regular discrete-time Fourier transform of the sequence x[n + m] w[m], where w[m] is a window sequence. It is also useful to define a time-dependent autocorrelation function for the sequence x[n] such that, for fixed n, its regular Fourier transform is the magnitude squared of the time-dependent Fourier transform. Specifically, the time-dependent autocorrelation function is defined as?
where X[n, ?) is defined by Eq. (10.18).
(a) Show that if x[n] is real?
i.e., for fixed n, c[n, m] is the a periodic autocorrelation of the sequence x[n + r]w[r], ??
(b) Show that the time-dependent autocorrelation function is an even function of m for n fixed, and use this fact to obtain the equivalent expression?
where
(c) What condition must the window w[r] satisfy so that Eq. (P10.39-1) can be used to compute c[n, m] for fixed m and ??
(d) Suppose that?
Find the impulse response hm[r] for computing the mth autocorrelation lag value, and find the corresponding system function Hm(z). From the system function, draw the block diagram of a causal system for computing the mth autocorrelation lag value c[n, m] for ??
(e) Repeat Part (d) for
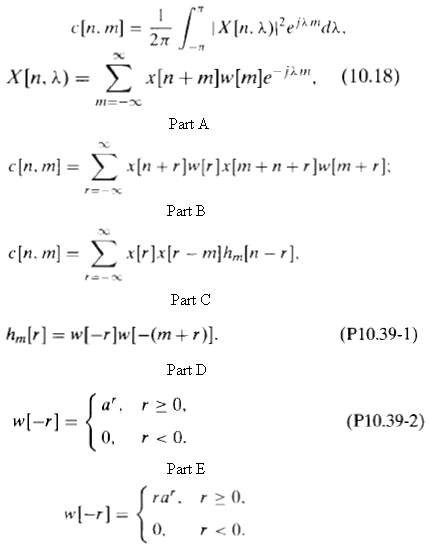
Step by Step Answer:

Discrete Time Signal Processing
ISBN: 978-0137549207
2nd Edition
Authors: Alan V. Oppenheim, Rolan W. Schafer





