Let x [n] and y [n] denote complex sequences and X(e j? ) and Y(e j? )
Question:
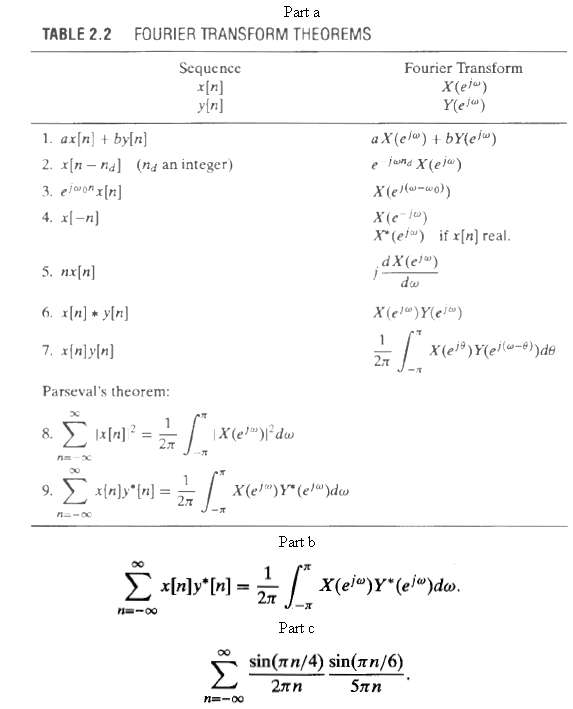
Let x [n] and y [n] denote complex sequences and X(ej?) and Y(ej?) their respective Fourier transforms.
(a) By using the convolution theorem (Theorem 6 in Table 2.2) and appropriate properties from Table 2.2, determine, in terms of x[n] and y[n], the sequence whose Fourier transform is X(ej?) Y*(ej?).
(b) Using the result in (a), show that Equation (P2.77-1) is a more general form of Parseval?s theorem, as given in section 2.9.5.
(c) Using Eq. (p2.77-1), determine the numerical value of the sum
Transcribed Image Text:
Part a TABLE 2.2 FOURIER TRANSFORM THEOREMS Fourier Transform X(el") Y(ej") Sequence x[n] yln] a X (elu) + bY(elu) e juna X(eja) 1. ax[n] + by[n] 2. x[n – na] 3. elaon I(n] (ng an integer) X(e (wmw) 4. x|-n) X(e u) X* (ei") if r[n] real. dX(el) 5. пx[n] dw 6. x[n] + y[n] X(e")Y(ei) X(e)Y(el(w-e))de 7. x(n]y[n] 27 Parseval's theorem: 8. Ix[n]? |X(e")dw 2л 9. x{n]y*[n] = X(e")Y" (e ")dw 27 n=-00 Part b * x(ei")Y*(ei)do. 2л E x[n]y*[n] = n=-00 Part c Σ sin(an/4) sin(rn/6) 5лп n=-00
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 70% (10 reviews)
a b c The Fourier transfor...View the full answer

Answered By

JAPHETH KOGEI
Hi there. I'm here to assist you to score the highest marks on your assignments and homework. My areas of specialisation are:
Auditing, Financial Accounting, Macroeconomics, Monetary-economics, Business-administration, Advanced-accounting, Corporate Finance, Professional-accounting-ethics, Corporate governance, Financial-risk-analysis, Financial-budgeting, Corporate-social-responsibility, Statistics, Business management, logic, Critical thinking,
So, I look forward to helping you solve your academic problem.
I enjoy teaching and tutoring university and high school students. During my free time, I also read books on motivation, leadership, comedy, emotional intelligence, critical thinking, nature, human nature, innovation, persuasion, performance, negotiations, goals, power, time management, wealth, debates, sales, and finance. Additionally, I am a panellist on an FM radio program on Sunday mornings where we discuss current affairs.
I travel three times a year either to the USA, Europe and around Africa.
As a university student in the USA, I enjoyed interacting with people from different cultures and ethnic groups. Together with friends, we travelled widely in the USA and in Europe (UK, France, Denmark, Germany, Turkey, etc).
So, I look forward to tutoring you. I believe that it will be exciting to meet them.
3.00+
2+ Reviews
10+ Question Solved
Related Book For 

Discrete Time Signal Processing
ISBN: 978-0137549207
2nd Edition
Authors: Alan V. Oppenheim, Rolan W. Schafer
Question Posted:
Students also viewed these Telecommunication Engineering questions
-
In working capital management, a financial manager needs to consider: I. accounts payable II. common stock III. fixed assets IV. a long-term debt a. II and IV only b. I only c. I, II, and IV only d....
-
Determine the signal f(t) whose Fourier transform is shown in Fig. 18.38. (Hint: Use the duality property.) F(a) 10 F 2 1 02
-
Describe what is meant by the term balanced in the term balanced scorecard method.
-
Suppose 1.0 cubic meter of oil is spilled into the ocean. Find the area of the resulting slick, assuming that it is one molecule thick, and that each molecule occupies a cube 0.50 m on a side.
-
Let (x) = 3x 2 - 4 and g(x) = x 2 - 3x - 4. Find each of the following. (g)(x)
-
Identificar las cuatro redes sociales principales y la forma en la que los gerentes de marca las integran en las acciones de marketing de sus organizaciones. OA3
-
True, False, or Uncertain: Countries with common-law based legal systems have relatively weak protections against investor expropriation.
-
Problem Threes A company reports the following amounts at the end of Year 1 (belore adjustment)
-
In Florida sunscreen and sunglasses are vital items If the tax on sellers of these items is doubled from 5.5 percent to 11 percent who will pay most of the tax increase the buyer or the seller. Will...
-
By applying the Fourier synthesis equation (Eq. (2.133)) to Eq. (2.172) and using Theorem 3 in Table 2.2, demonstrate the validity of the modulation theorem (Theorem 7, Table 2.2). FOURIER TRANSFORM...
-
Let x[n] and X(e j ) represent a sequence and its Fourier transform, respectively. Determine in terms of X(e j ), the transforms of y s [n], y d [n], and y e [n] In each case, sketch y(e j ) for X(e...
-
From Concept Check 4, record the May 31 semiannual payment and amortization of Premium on Bonds Payable. In Concept Check 4, Redo Concept Check 2 with the straight-line method, assuming that the bond...
-
1. Using appropriate examples, compare and contrast the genetic diversity of marine fish species with freshwater fish species (8 marks) 2. Your class went on a trip and discovered a crater lake on...
-
Find sin(29) given that cos(0) = and 0
-
Amazing Aquariums began as a class project on new business development. Now that the visionaries behind the idea have graduated, they want to explore their business idea and see if the concept could...
-
3. Modify the program of Example 05 so that, it takes and prints values using the following two functions respectively. void get (double *&a, int& n); void print (double *a, int n); 4. Following is a...
-
A company will be financing its operations with and a capital budget is P40,000,000 and a debt-to-equity ratio of 1. The interest rate on company's debt is 10%. The expected return on equity by the...
-
What can you do to ease your familys transition? What support relationships will you have to build? Which are your highest priorities? AppendixLO1
-
What is your opinion of advertising awards, such as the Cannes Lions, that are based solely on creativity? If you were a marketer looking for an agency, would you take these creative awards into...
-
(a) Find the total Coulomb force on a charge of 2.00 nC located at x = 4.00 cm in Figure 18.48 (b), given that q = 1.00 C. (b) Find the x-position at which the electric field is zero in Figure 18.48...
-
Determine the minimum-phase system whose squared magnitude response is givenby: (a) H(= cos to 21 - a) (i + a) - 2a cos s (h) Him): al < 1
-
Consider an FIR system with the following system function:? H(z) = (1 ? 0.8 e j?/2 z -1 )(1 ? 0.8 e- j?/2 z -1 ) (1 ? 1.5 e j?/4 z -1 ) (1 ? 1.5 e- j?/4 z -1 ) (a) Determine all systems that have the...
-
The causal system is known to be unstable. We modify this system by changing its impulse response h(n) to h(n) = n h(n)u(n). (a) Show that by properly choosing we can obtain a new stable system....
-
Compute the value of ordinary bonds under the following circumstances assuming that the coupon rate is 0.06:(either the correct formula(s) or the correct key strokes must be shown here to receive...
-
A tax-exempt municipal bond has a yield to maturity of 3.92%. An investor, who has a marginal tax rate of 40.00%, would prefer and an otherwise identical taxable corporate bond if it had a yield to...
-
Please note, kindly no handwriting. Q. Suppose a 3 year bond with a 6% coupon rate that was purchased for $760 and had a promised yield of 8%. Suppose that interest rates increased and the price of...

Study smarter with the SolutionInn App


