X[n] and y[n] are two real-valued, positive, finite-length sequences of length 256; i.e.,?

x[n] > 0, 0 ? n ? 225,

y[n] > 0, 0 ? n ? 255,

x[n] = y[n] = 0, otherwise

r[n] denotes the linear convolution of x[n] and y[n]. R(e^j?) denotes the Fourier transform of r[n]. R_s[k] denotes 128 equally spaced samples of R(e^j?); i.e.,

R_s[k] R(e^j?)|w=2?k/128,? ? ? ? ?k = 0. 1,?., 127.

Given x[n] and y[n], we want to obtain R_s[k] as efficiently as possible. The only modules available are those shown in Figure. The costs associated with each module are as follows:

Modules I and II are free.

Module III costs 10 units.

Module IV costs 50 units.

Module V costs 100 units.?

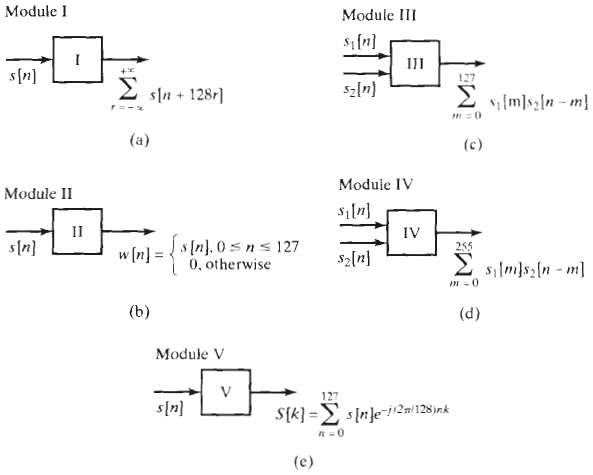
By appropriately connecting one or several of each module, construct a system for which the inputs are x[n] and y[n] and the output is R_s[k]. The important considerations are (a) whether the system works and (b) how efficient it is. The lower the total cost, the more efficient the system is.

Module I Module III $i{n] III s[n] 127 szln) s[n + 128r] ;[m]s2{n - m} (a) (c) Module IV Module II si[n] II IV s [n]. 0 s ns 127 0, otherwise s[n] 255 w (n] = $2[n} 2 slm}sz[n - m] m -0 (b) (d) Module V 127 s[n] S[k] = sIr]e2/128)nk n-0 (e)