Since the variances of the mean and the midrange are not affected if the same constant is added to each observation, we can determine these variances for random samples of size 3 from the uniform population

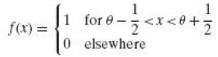
By referring instead to the uniform population

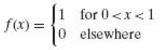
(a) Show that E(X) = 1/2 , E(X²) = 1/3 , and var(X) = 1/12 for this population so that for a random sample of size n = 3, var(X) = 1/36 .

(b) Use the results of Exercises 8.46 and 8.52 on page 254 (or derive the necessary densities and joint density) to show that for a random sample of size n = 3 from this population, the order statistics Y₁ and Y₃ have E(Y₁) = 14 , E(Y²₁) = 1/10 , E(Y₃) = 34 , E(Y²₃) = 35 , and E(Y₁Y₃) = 1/5 so that var(Y₁) = 3/80 , var(Y₃) = 3/80, and cov(Y₁,Y₃) = 1/80.

(c) Use the results of part (b) and Theorem 4.14 on page 135 to show that E Y1 + Y3 2 = 1 2 and var(Y₁ + Y₃ / 2) = 1 40 and hence that for random samples of size n = 3 from the given uniform population, the midrange is unbiased and more efficient than the mean.

Transcribed Image Text:

for 2 f(x) = 0 elsewhere 1 for 0r1 elsewhere f(x)=10