Show that for a field F, the set S of all matrices of the form for a, b F is a right ideal but not a left ideal of M 2 (F) That is, show that S is a subring closed under multiplication on the right by any element of M 2 (F), but is not closed under left multiplication b (86) 0 0...

Clearly S is closed under addition contains the zero matrix and contains the additive i ...

Show that for a field F, the set S of all matrices of the form for a,

Question:

Show that for a field F, the set S of all matrices of the form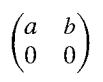

for a, b ∈ F is a right ideal but not a left ideal of M₂(F). That is, show that S is a subring closed under multiplication on the right by any element of M₂(F), but is not closed under left multiplication.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

A First Course In Abstract Algebra

ISBN: 9780201763904

7th Edition

Authors: John Fraleigh

See More Books

Question Posted: Jan 05, 2023 05:26 AM

See More Questions

Show that for a discrete uniform random variable X, if each of the values in the range of X is multiplied by the constant c, the effect is to multiply the mean of X by c and the variance of X by c2....
Show that for a van der Waals gas, Cp,m Cv,m = R 1/ = 1 (3Vt 1)2/4V3tTt and evaluate the difference for xenon at 25C and 10.0 atm.
Show that for a constant acceleration, 2
Determine the number of valence electrons for each of thefollowing four elements. Part A Ga _______ Express your answer as an integer. Part B Pb ________ Express your answer as an integer. Part C Cl...
(a) In question 16 what advanced statistical procedures can Poindexter employ to improve his predictions about test errors even more? (b) Say that the resulting correlation coefficient is .67. Using...
Figure P15.10 is a snapshot graph of a wave at $t=2 \mathrm{~s}$. Draw the history graph for this wave at $x=0 \mathrm{~m}$, for $t=0 \mathrm{~s}$ to $8 \mathrm{~s}$. y (cm) 1 m/s- 1 2 3 4 5...
What are the Fourth and Seventh Directives? LO4
In the past, Arup Mukherjees tire dealership in Pensacola sold an average of 1,000 radials each year. In the past 2 years, 200 and 250, respectively, were sold in fall, 350 and 300 in winter, 150 and...
Discuss the major implications of health reform on financial management of healthcare organizations. In your opinion, what aspects of the Affordable Care Act should be maintained, which should be...
Why is the iPhone considered a disruptive innovation?
Referring to Exercise 34, show by examples that for proper ideals N of a commutative ring R, a. N need not equal N b. N may equal N. Data from Exercise 34 Let R be a commutative ring and N an ideal...
Referring to Exercise 32, give an example of a subset S of R 2 such that V(I(S)) S. Data from Exercise 32 Let F be a field. Show that if S is a nonempty subset of F n , then I(S) = {f(x) F[x]|f(s)...
Some people-usually business travelers-have a very strong desire to fly to a particular city on a particular day, and airlines charge these travelers higher ticket prices than they charge other...
Consider the following C functions and assembly code: int fun4 (int *ap, int *bp) ( int a = *ap; int bbp; return a+b; }) pushl ebp movl esp, ebp int fun5 (int *ap, int *bp) { int bbp; *bp + *ap;...
The position of a particle moving along the x-axis is given by x(t) = = 4.2 2.5t m. (Assume t is in seconds.) (a) At what time (in s) does the particle cross the origin? 1.68 S (b) What is the...
2. Boxes A and B are being pulled to the right by a rope attached to box B. Box A sits on top of box B, and both boxes accelerate together to the right at a rate of 1.75 m/s. The masses and...
You bought a 15-kilogram sack of unshelled peanuts for your restaurant. You weigh the sack three times on a balance, with the following results: Trial Mass (kg) 1 15.02 2 15.49 3 15.91 The results...
Two hikers leave the same tent at a campground and go separate ways. One hiker walks 8 miles directly south to Ashville, and the other hiker walks 14 miles directly northwest (i.e., N45W) to...
Assume that a sample of bivariate data yields the correlation coefficient, r, indicated. Use Table 12.9 for the specified sample size and level of significance to determine whether a linear...
The production budget of Artest Company calls for 80,000 units to be produced. If it takes 30 minutes to make one unit and the direct labor rate is $16 per hour, what is the total budgeted direct...
During the 1999 tax filing season, 13.7% of all individual U.S. tax returns were prepared by H&R Block. For a random selection of 3 tax returns, describe the probability distribution for x = the...
It has been estimated that 40% of all U.S. children are covered by life insurance. For a randomly selected group of 10 children, use the appropriate statistical table to describe the probability...
It has been estimated that about 30% of families with an annual income of $100,000 or more own savings bonds. What is the probability that exactly three of a randomly selected group of five such...
1,600 Balance Sheet The following is a list (in random order) of KIP International Products Company's December 31, 2019, balance sheet accounts: Additional Paid-In Capital on Preferred Stock $2,000...
Question 3 4 pts 9 x + 3 x 9 if x 0 Find a) lim f(x), b) lim, f(x), C), lim , f(x) if they exist. 3 Edit View Insert Format Tools Table : 12pt M Paragraph B IV A2 Tv
Mr. Geoffrey Guo had a variety of transactions during the 2019 year. Determine the total taxable capital gains included in Mr. Guo's division B income. The transactions included: 1. On January 1,...

Show that for a field F, the set S of all matrices of the form for a,

Question:

b (86) 0 0

Step by Step Answer:

Clearly S is closed under addition contains the zero matrix and contains the additive i...View the full answer

A First Course In Abstract Algebra

Students also viewed these Mathematics questions