Every torsion-free divisible abelian group D is a direct sum of copies of the rationals Q. if
Question:
Every torsion-free divisible abelian group D is a direct sum of copies of the rationals Q. if O ≠ n e Z and α ϵ D, then there exists a unique b ϵ D such that nb = a. Denote h by (1/n)a. For m, n ϵ Z (n ≠ 0), define (m/n)a = m(1/n)a. Then D is a vector space over Q. Use Theorem 2.4.
Data from Theorem 2.4
Every vector space V over a division ring D has a basis and is therefore a free D-module. More generally every linearly independent subset of V is contained in a basis of V. The converse of Theorem 2.4 is also true, namely, if every unitary module over a ring D with identity is free, then D is a division ring (Exercise 3.14).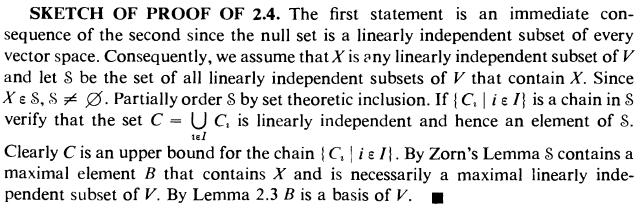
Data from Exercise 3.14
Let R be a commutative ring with identity and prime characteristic p. The map R →R given by r |→ rP is a homomorphism of rings called the Frobenius homomorphism [see Exercise 11 ].
Data from Exercise 11
(The Freshman's Dream1). Let R be a commutative ring with identity of prime characteristic p. If a,b ϵ R, then![]() for all integers n > 0 [see
Theorem 1.6 and Exercise 10; note that b = -b if p = 2].
for all integers n > 0 [see
Theorem 1.6 and Exercise 10; note that b = -b if p = 2].
Data from Theorem 1.6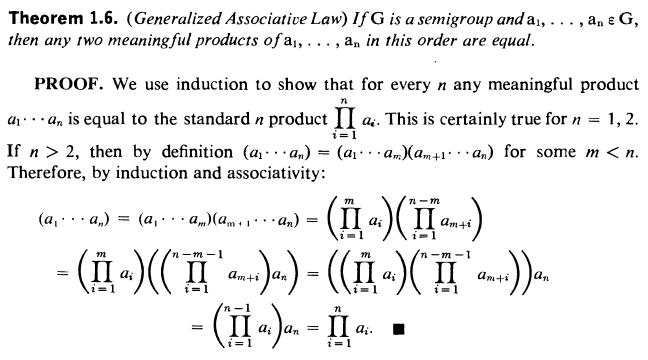 Data from Exercise 10
Data from Exercise 10
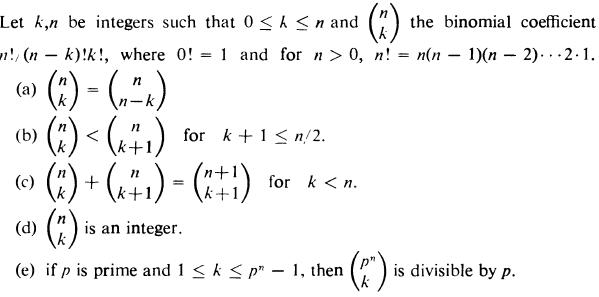
Step by Step Answer:

Algebra Graduate Texts In Mathematics 73
ISBN: 9780387905181
8th Edition
Authors: Thomas W. Hungerford





