If c_o, c₁, ... , C_n are distinct elements of an integral domain D and d_o, ... , d_n are any elements of D, then there is at most one polynomial ∫ of degree ≤ n in D[x] such that ∫(c_i) = d_i for i = 0, 1, ... ,n. [For the existence of ∫, see Exercise 12].

Data from exercise 12

Lagrange's Interpolation Formula. If F is a field, a₀,a₁, ... , a_n are distinct elements of F and c₀,c₁, ... , C_n are any elements of F, then 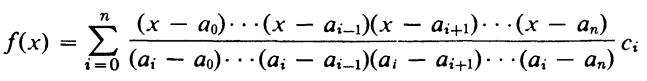is the unique polynomial of degree ≤ n in F[x] such that ∫(a_i) = C_i for all i 

Transcribed Image Text:

f(x) − … . @i_l)(x ao). - = 2 (ax = a) (x -at-1)(x - ai+1). (Can) . . ai_1)(ai - - i=0 . . . Ci