Let R be a commutative ring with identity and let ∫,g e R[[xJJ. Denote by In ∫, the "' initial degree of ∫ (that is, the smallest n such that a_n ≠  0, where 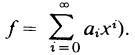Show that

(a) In (∫ + g) ≥ min (In ∫, In g).

(b) In (∫g) ≥ In ∫ + In g.

(c) If R is an integral domain, In (∫g) = In ∫ + In g. 

Transcribed Image Text:

f= Σ dixi). i=0