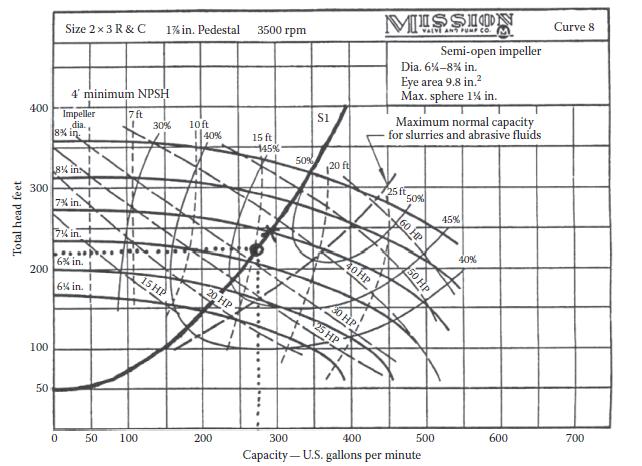
Water at $60^{\circ} \mathrm{F}$ is to be transferred at a rate of $250 \mathrm{gpm}$ from the bottom of a storage tank to the bottom of a process vessel. The water level in the storage tank is $5 \mathrm{ft}$ above ground level, and the pressure in the tank is $10 \mathrm{psig}$. In the process vessel, the level is $15 \mathrm{ft}$ above ground and the pressure is 20 psig. The transfer line is $150 \mathrm{ft}$ of 3 in. sch 40 pipe, containing eight flanged elbows, three $80 %$ reduced trim gate valves, and a $3 \times 3$ control valve. The pump in the line has the same characteristics as those shown in Figure 8.2 with an 8 in. impeller, and the control valve has a linear characteristic. If the stem on the control valve is set to provide the desired flow rate under the specified conditions, what should be the required value of the valve coefficient to achieve this?

Figure 8.2

Transcribed Image Text:

Size 23 R & C 1% in. Pedestal 3500 rpm 4' minimum NPSH 400 Impeller Total head feet 8% in 300 200 8% ins 7% in 7% in 6% in. 6% in. 1 100 50 0 S1 MISSION VALVE AND PUMP CO. M Semi-open impeller Dia. 6%-8% in. Eye area 9.8 in. Max. sphere 1% in. Maximum normal capacity for slurries and abrasive fluids Curve 8 7 ft 30% 10ft 40% 15 ft 145% 50% 20 f 25 ft 50% 45% 15 HP 20 HP 50 100 200 300 40HP 30 HP 25 HP 60 HP 50 HP 400 40% 500 600 700 Capacity-U.S. gallons per minute