In Example 2, change the denominator of the third fraction to 4a²b² and then find the LCD.

Data from Example 2

Find the LCD of the fractions

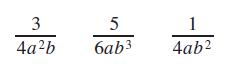
We first express each denominator in terms of powers of its prime factors:

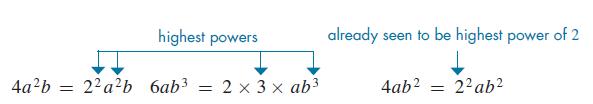
The prime factors to be considered are 2, 3, a, and b. The largest exponent of 2 that appears is 2. Therefore, 2² is a factor of the LCD. [What matters is that the highest power of 2 that appears is 2, not the fact that 2 appears in all three denominators with a total of five factors.] The largest exponent of 3 that appears is 1 (understood in the second denominator). Therefore, 3 is a factor of the LCD. The largest exponent of a that appears is 2, and the largest exponent of b that appears is 3. Thus, a² and b³ are factors of the. Therefore, the LCD of the fractions is 2² × 3 × a²b³ = 12a²b³

This is the simplest expression into which each of the denominators above will divide exactly.

3 4ab 5 6ab3 1 4ab