In Example 5, change $700 to $830 and then solve for the values of the investments.

Data from Example 5

Two investments totaling $18,000 yield an annual income of $700. If the first investment has an yield rate of 5.5% and the second a rate of 3.0%, what is the value of each?

Let x = the value of the first investment and y = the value of the second investment. We know that the total of the two investments is $18,000. This leads to the equation x + y = $18, 000. The first investment yields 0.055x dollars annually, and the second yields 0.030y dollars annually. This leads to the equation 0.055x + 0.030y = 700. These two equations are then solved simultaneously:

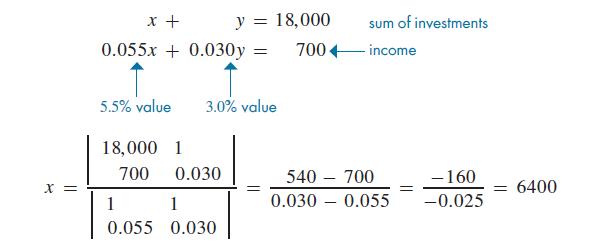
The value of y can be found most easily by substituting this value of x into the first equation, y = 18,000 − x = 18,000 − 6400 = 11,600.

Therefore, the values invested are $6400 and $11,600. Checking, the total income is $6400(0.055) + $11,600(0.030) = $700, which agrees with the statement of the problem.

X = x + 0.055x+ 0.030y 18,000 1 700 5.5% value 3.0% value 1 0.055 y = 18,000 700 0.030 1 0.030 030 = sum of investments income 540 700 0.030 0.055 - = - 160 -0.025 = 6400