Make the given changes in the indicated examples of this section, and then solve the resulting problems.
Question:
Make the given changes in the indicated examples of this section, and then solve the resulting problems.
In Example 6, change 2.00 s to 2.50s.
Data from Example 6
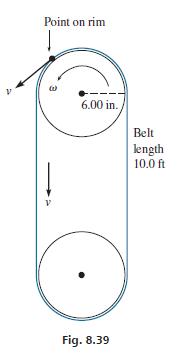
A pulley belt 10.0 ft long takes 2.00 s to make one complete revolution. The radius of the pulley is 6.00 in. What is the angular velocity (in revolutions per minute) of a point on the rim of the pulley? See Fig. 8.39.
Because the linear velocity of a point on the rim of the pulley is the same as the velocity of the belt, v = 10.0 ft/2.00 s = 5.00 ft/s. The radius of the pulley is r = 6.00 in. = 0.500 ft, and we can find ω by substituting into Eq. (8.11). This gives us
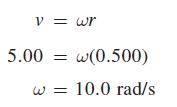
To convert this answer to revolutions per minute, we use the procedure described in Section 1.4:
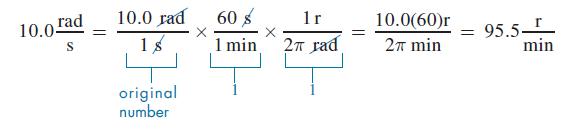
Step by Step Answer:

Basic Technical Mathematics
ISBN: 9780137529896
12th Edition
Authors: Allyn J. Washington, Richard Evans





