Suppose that x has a Poisson distribution (see question 6) P() of mean and that, for

Suppose that x has a Poisson distribution (see question 6) P(λ) of mean λ and that, for given x, y has a binomial distribution B(x,π) of index x and parameter π.

(a) Show that the unconditional distribution of y is Poisson of mean 

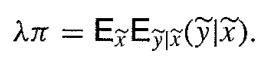
(b) Verify that the formula 

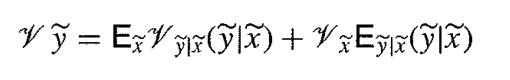
derived in Section 1.5 holds in this case.

Question 6

Suppose that k ~ B(n,π) where n is large and π is small but nπ  = λ has an intermediate value. Use the exponential limit ( 1 + x )ⁿ → e^x to show that P(k = 0) ≌ e^-λ and P(k = I) ≌  λe^-λ . Extend this result to show that k is such that 

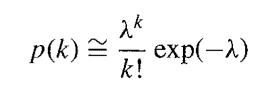
that is, k is approximately distributed as a Poisson variable of mean λ.

Transcribed Image Text:

λπ = ΕΕ, (3|3).