Setting the right price for a product is an important business decision. If the product is priced too high, the demand could be very low.

Set the price too low, demand may be high, but we are potentially leaving money on the table because the revenue per unit is low. Pricing analytics involves finding the right tradeoff between price charged and demand so as to maximize revenue. Suppose we need to set the price for renting a subcompact automobile for one day. Let us outline the decision process:

Step 1. Identify and define the problem. We need to set a price per day for a midsize rental car.

Step 2. Determine the criteria that will be used to evaluate alternative solutions.

Our goal in setting the price is to maximize revenue per day.

Step 3. Determine the set of alternative solutions. Based on historical data and the competition, we will consider a broad price range from \($10\) per day to \($60\) per day per car.

Step 4. Evaluate the alternatives. We will evaluate proposed prices based on the expected revenue per day.

Step 5. Choose an alternative. We will choose the price that maximizes expected revenue.

We can use data and analytics to complete steps 4 and 5 of the decision process.

a. Based on historical or test-market data, we can estimate a model that gives expected revenue as a function of price, as shown below. The dots represent the data (price and demand combinations) and the estimated model is the line in the chart: Demand -1.4028(Price) + 102.65. For example, for price of \($35\), Demand = -1.4028(35) + 102.65 = 53.552 vehicles. So, we estimate that at a price of \($35\) per day, the demand will be about 54 vehicles. Is this estimated equation, a descriptive, predictive, or prescriptive model? Explain.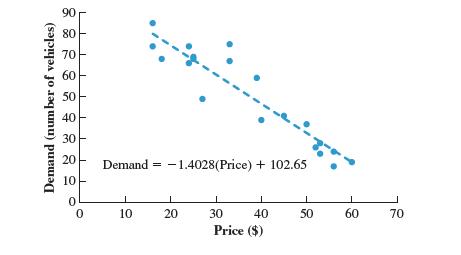

Our goal (step 5) is to find the price that maximizes expected revenue. Revenue = demand X price which is (-1.4028(Price) + 102.65) X (Price) = -1.40228(Price) + 102.65(Price). The revenue as a function of price is shown below for \($10\) increments of price.

b. What is the price that maximizes revenue?

c. Is step 5, visually inspecting the revenue function to find a revenue-maximizing price, descriptive, predictive, or prescriptive analytics? Explain.

Transcribed Image Text:

Demand (number of vehicles) 90 80 70 60 50 40 T T 30- 20 Demand = -1.4028(Price) + 102.65 10 0 0 10 20 30 40 50 Price ($) 60 60 70